Chapter 10 Congruent Triangles RD Sharma Solutions Exercise 10.2 Class 9 Maths

Chapter Name | RD Sharma Chapter 10 Congruent Triangles Exercise 10.2 |
Book Name | RD Sharma Mathematics for Class 10 |
Other Exercises |
|
Related Study | NCERT Solutions for Class 10 Maths |
Exercise 10.2 Solutions
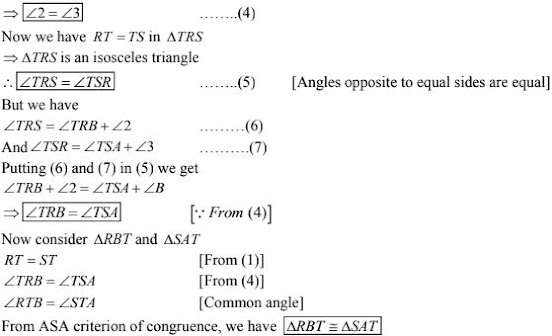
1. In Fig. 10.40, it is given that RT = TS, ∠1 = 2∠2 and ∠4 = 2∠3. Prove that ΔRBT ≅ ΔSAT.
Solution

In the figure given that
RT = TS ...(1)
∠1 = 2∠2 ...(2)
and ∠4 = 2∠3 ...(3)
and given to prove ΔRBT ≅ ΔSAT
Let the point of intersection of RB and SA be denoted by O
Since RB and SA intersect at O.
∴ ∠AOR = ∠BOS [Vertically opposite angles]
⇒ ∠1 = ∠4
⇒ 2∠2 = 2∠3 [From (2) and (3)]
2. Two lines AB and CD intersect at O such that BC is equal and parallel to AD. Prove that the lines AB and CD bisect at O.
Solution

Given that lines AB and CD intersect at O
Such that BC || AD and BC = AD ...(1)
We have to prove that AB and CD bisect at O.
To prove this first we have to prove that ΔAOD ≅ ΔBOC
3. BD and CE are bisectors of ∠B and ∠C of an isosceles ΔABC with AB = AC. Prove that BD = CE.
Solution

Given that ΔABC is isosceles with AB = AC and BD and CE are bisectors of ∠B and ∠C
We have to prove BD = CE
Since, AB = AC
⇒ ∠ABC = ∠ACB ...(1)
[∵ Angles opposite to equal sides are equal]
Since BD and CE are bisectors of ∠B and ∠C
⇒ ∠ABD = ∠DBC = ∠BCE = ECA = ∠B/2 = ∠C/2 ...(2)
Now,
Now,
Consider ΔEBC and ΔDCB
∠EBC = ∠DCB [∵ ∠B = ∠C] from (1)
BC = BC [Common side]
BC = BC [Common side]


