RD Sharma Solutions for Class 10 Chapter 15 Areas Related to Circles Exercise 15.2

Chapter 15 Areas Related to Circles RD Sharma Solutions Exercise 15.2 Class 10 Maths

Chapter Name	RD Sharma Chapter 15 Areas Related to Circles
Book Name	RD Sharma Mathematics for Class 10
Other Exercises	Exercise 15.1 Exercise 15.3 Exercise 15.4
Related Study	NCERT Solutions for Class 10 Maths

Exercise 15.2 Solutions

1. Find in terms of x the length of the arc that subtends an angle of 30° , at the centre of circle of radius 4 cm. 

Solution

2. Find the angle subtended at the centre of circle of radius 5cm by an arc of length (5π/3) cm.

Solution

Radius(r) = 5cm 

3. An arc of length 20π cm subtends an angle of 144° at centre of circle. Find the radius of the circle. 

Solution

4. An arc of length 15cm subtends an angle of 45° at the centre of a circle. Find in terms of π, radius of the circle. 

Solution

5. Find the angle subtended at the centre of circle of radius  'a' cm by an arc of length aπ/4 cm 

Solution

6. A sector of circle of radius 4cm contains an angle of 30° . Find the area of sector.

Solution

 7. A sector of a circle of radius 8cm contains the angle of 135° . Find the area of sector. 

Solution

8. The area of sector of circle of radius 2cm is πcm². Find the angle contained by the sector. 

Solution

9. The area of sector of circle of radius 5cm is 5πcm² . Find the angle contained by the sector. 

Solution

10. AB is a chord or circle with centre O and radius 4cm. AB is length of 4cm. Find the area of sector of the circle formed by chord AB 

Solution

11. In a circle of radius 35cm, an arc subtends an angle of 72° at the centre. Find the length of arc and area of sector. 

Solution

12. The perimeter of a sector of circle of radius 5.7m is 27.2m. Find the area of sector. 

Solution

13. The perimeter of certain sector of circle of radius 5.6 m is 27.2 m. Find the area of sector. 

Solution

14. A sector is cut - off from a circle of radius 21 cm the angle of sector is 120°. Find the length of its arc and its area. 

Solution

15. The minute hand of a clock is √21 cm long. Find area described by the minute hand on the face of clock between 7am and 7:05 am 

Solution

16. The minute hand of clock is 10cm long. Find the area of the face of the clock described by the minute hand between 8 am and 8:25 am 

Solution

17. A sector of 56° cut out from a circle contains area of 4.4 cm² . Find the radius of the circle 

Solution

Angle subtended by sector at centre θ = 56° 

Let radius be 'x' cm 

18. In circle of radius 6cm, chord of length 10cm makes an angle of 110° at the centre of circle find 

(i) Circumference of the circle 

(ii) Area of the circle 

(iii) Length of arc 

(iv) The area of sector 

Solution

(i) Radius of circle (r) = 6cm 

Angle subtended at the centre = 110°
Circumference of the circle = 2πr 

19. Below fig. shows a sector of a circle, centre O containing an angle θ° Prove that 

(i) Perimeter of shaded region is r(tan θ + sec θ + πθ/180 - 1)
(ii) Area of shaded region is r²(tan θ - πθ/180) 

Solution

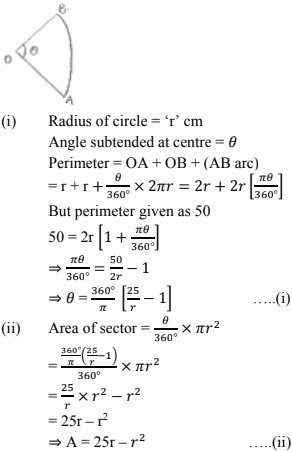
20. The diagram shows a sector of circle of radius 'r' can containing an angle θ. The area of  sector is A cm² and perimeter of sector is 50 cm. Prove that 

(i) θ = 360/π(25/r - 1) 
(ii) A = 25r - r².

Solution