RD Sharma Solutions for Class 10 Chapter 15 Areas Related to Circles Exercise 15.1

Chapter 15 Areas Related to Circles RD Sharma Solutions Exercise 15.1 Class 10 Maths

Chapter Name	RD Sharma Chapter 15 Areas Related to Circles
Book Name	RD Sharma Mathematics for Class 10
Other Exercises	Exercise 15.2 Exercise 15.3 Exercise 15.4
Related Study	NCERT Solutions for Class 10 Maths

Exercise 15.1 Solutions

1. Find the circumference and area of circle of radius 4.2 cm .

Solution

Radius (r) = 4.2 cm 
Circumference = 2 × r 

2. Find the circumference of a circle whose area is 301.84 cm² .

Solution

Area of circle  = 301.84cm² .

Let radius =  r cm 

Area of circle = πr² 

3. Find the area of circle whose circumference is 44 cm.

Solution

Circumference = 44 cm 

Let radius = r cm 

Circumference = 2 × r = 44 cm

4. The circumference of a circle exceeds diameter by 16.8 cm. Find the circumference of circle.

Solution

Let radius of circle = r cm

Diameter (d) = 2× radius  = 2r 
Circumference (c) = 2πr 
Given circumference exceeds diameter by 16.8 cm 

5. A horse is tied to a pole with 28m long string. Find the area where the horse can graze. 

Solution

Length of string l = 28m

6. A steel wire when bent is the form of square encloses an area of 12 cm² . If the same wire is bent in form of circle. Find the area of circle. 

Solution

7. A horse is placed for grazing inside a rectangular field 40 m by 36 m and is tethered to one corner by a rope 14m long. Over how much area can it graze. 

Solution

The fig shows rectangular field ABCD at corner A, a horse is tied with rope length = 14 m. 

The area it can graze is represented A as shaded region = area of quadrant with (radius = length) of string

8. A sheet of paper is in the form of rectangle ABCD in which AB = 40 cm and AD = 28 cm.

A semicircular portion with BC as diameter is cut off. Find the area of remaining paper. 

Solution

9. The circumference of two circles are in ratio 2 : 3. Find the ratio of their areas 

Solution 

Let radius of two circles be r₁ and r₂ then their circumferences will be 2πr₁ : 2πr₂ = r₁ : r₂ 
But circumference ratio is given as 2 : 3 
 r₁ : r₂ = 2 : 3 

10. The side of a square is 10 cm. find the area of circumscribed and inscribed circles. 

Solution

Circumscribed circle 

11. The sum of the radii of two circles is 140 cm and the difference of their circumferences in 88 cm. Find the diameters of the circles. 

Solution

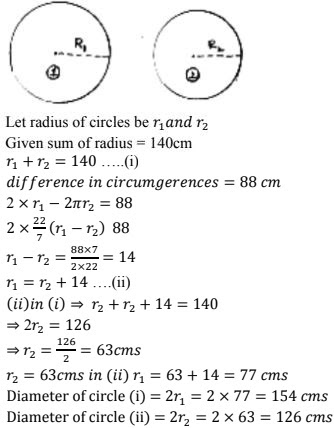
12. The area of circle, inscribed in equilateral triangle is 154 cm² . Find the perimeter of triangle. 

Solution

13. A field is in the form of circle. A fence is to be erected around the field. The cost of fencing would to Rs. 2640 at rate of Rs. 12 per metre. Then the field is to be thoroughs ploughed at cost of Rs. 0.50 per m² . What is amount required to plough the field ? 

Solution

Given,

Total cost of fencing the circular field = Rs. 2640 
Cost per metre fencing = Rs. 12 
Total cost of fencing = circumference × cost per fencing 

⇒2640 = circumference × 12 
⇒ circumference = 2640/12 = 220 m 

Let radius of field be r m 

Circumference = 2πr m 

2πr = 220 
2 × 22/7 × r = 220 
r = 70/2 = 35m

Area of field = πr² .

= 22/7 × 35×35 
= 3850 m² .

Cost of ploughing per m² land = Rs. 0.50 

Cost of ploughing 3850 m² land = 1/2 × 3850 = Rs. 1925. 

14. If a square is inscribed in a circle, find the ratio of areas of the circle and the square. 

Solution

15. A park is in the form of rectangle 120m × 100m. At the centre of park there is a circular lawn. The area of park excluding lawn is 8700m² . Find the radius of circular lawn. 

Solution

16. The radii of two circles are  8cm and 6cm respectively. Find the radius of the circle having its are equal to the sum of the areas of two circles. 

Solution

Radius of circles are 8 cm and 6 cm 

17. The radii of two circles are 19cm and 9 cm respectively. Find the radius and area of the circle which has circumferences is equal to sum of circumference of  two circles. 

Solution

Radius of 1st circle = 19cm 

Radius of 2nd circle = 9cm 

Circumference of 1st circle = 2(19) = 38π cm 

Circumference of 2nd circle = 2π(9) = 18π cm 

Let radius of required circle = R cm 

18. A car travels 1 km distance in which each wheel makes 450 complete revolutions. Find the radius of wheel. 

Solution

Let radius of wheel = 'r' m 

19. The area enclosed between the concentric circles is 770 cm² . If the radius of inner circle. 

Solution

Radius of outer circle = 21 cm