Chapter 18 Areas of Circle, Sector & Segment RS Aggarwal Solutions MCQ Class 10 Maths
Chapter Name | RS Aggarwal Chapter 18 Areas of Circle, Sector & Segment |
Book Name | RS Aggarwal Mathematics for Class 10 |
Other Exercises |
|
Related Study | NCERT Solutions for Class 10 Maths |
Areas of Circle, Sector & Segment MCQ Solutions
1. The area of a circle is 38.5 cm2. The circumference of the circle is
A. 6.2 cm
B. 12.1 cm
C. 11 cm
D. 22 cm
Solution
Let the radius if the circle be r
Given, Area of circle = 38.5 cm2
Area of circle = πr2
⇒ Ï€r2 = 38.5
Since π = 22/7
⇒ 22/7 × r2 = 38.5
⇒ r2 = 38.5 × (7/22)
⇒ r2 = 12.25
⇒ r = √12.25 = 3.5 cm
∴ Radius of circle = 3.5 cm
Circumference of circle = 2Ï€r
= 2 × 22/7 × 3.5 cm
= 22 cm
∴ Circumference of the circle is 22 cm
Let the radius if circle be r
Given, Area of circle = 38.5 cm2
Area of circle = πr2
Ï€r2 = 38.5
Since = 22/7
∴ Ï€r2 = 38.5
⇒ 22/7 × r2 = 38.5
⇒ r2 = 12.25
⇒ r = √12.25 = 3.50 cm
∴ Radius of circle = 3.5 cm
Circumference of circle = 2Ï€r
= 2 × 22/7 × 3.5
= 22 cm
∴ Circumference of the circle is 22 cm.
2. The area of a circle is 49Ï€ cm2. Its circumference is
A. 7Ï€ cm
B. 14Ï€ cm
C. 21Ï€ cm
D. 28Ï€ cm
Solution
Let the radius if circle be r
Given, Area of circle = 49Ï€ cm2
Area of circle = πr2
Ï€r2 = 49Ï€
Since = 22/7
∴ Ï€r2 = 49Ï€
⇒ r2 = 49
⇒ r = √49 = 7 cm
∴ Radius of circle = 7 cm
Circumference of circle = 2Ï€r
= 2 × r × 7 cm
= 14Ï€ cm
∴ Circumference of the circle is 14Ï€ cm.
3. The difference between the circumference and radius of a circle is 37 cm. The area of the circle is
A. 111 cm2
B. 84 cm2
C. 154 cm2
D. 259 cm2
Solution
Let the radius if circle be r
Circumference of circle = 2Ï€r
Difference between the circumference and radius of a circle = 37 cm
⇒ 2Ï€r – r = 37 cm
⇒ 2 × 22/7 × r – r = 37 cm
⇒ 44/7 × r – r = 37 cm
⇒ (44/7 – 1) × r = 37 cm
⇒ 37/7 × r = 37 cm
⇒ r = 37 × 7/37
⇒ r = 7 cm
Area of circle = πr2
= 22/7 × 7 × 7 cm2
= 22/7 × 49 cm2
= 22 × 7 cm2
= 154 cm2
∴ Area of the circle is 154 cm2
4. The perimeter of a circular field is 242 m. The area of the field is
A. 9317 m2
B. 18634 m2
C. 4658 m2
D. none of these
Solution
Let the radius if circular field be r
Perimeter of circular field = 2Ï€r
Perimeter of circular field = 242 m
⇒ 2Ï€r = 242 m
⇒ 2 × 22/7 × r = 242 m
⇒ r = 242 × 1/2 × 7/22 = 38.5 m
∴ Radius of circular field = 38.5 m
Area of the filed = πr2
= 22/7 × 38.52 m2
= 22/7 × 1482.5 m2 = 4658.5 m2
∴ Area of the field = 4658.5 m2
5. On increasing the diameter of a circle by 40%, its area will be increased by
A. 40%
B. 80%
C. 96%
D. 82%
Solution
Let the radius if circle be r
Area of circle = A = πr2
Radius increases by 40%
So, new radius r’ = r + 40/100 × r = 1.4r
New Area of circle = A’ = Ï€r’2 = Ï€ × (1.4r)2
= 1.96Ï€r2
Percentage increase in area = (A’ – A)/A × 100
= (1.96Ï€ r2 – Ï€r2)/Ï€r2 × 100
= 0.96 × 100
= 96
∴ Increase in area = 96%
6. On decreasing the radius of a circle by 30%, its area is decreased by
A. 30%
B. 60%
C. 45%
D. none of these
Solution
Let the radius if circle be r
Area of circle = A = πr2
Radius decreases by 30%
So, New Radius r’ = r – 30/100 × r = 0.7 r
New Area of circle = A’ = Ï€r’2 = Ï€ × (0.7r)2
= 0.49 πr2
Percentage decrease in area = (A – A’)/A × 100
= (Ï€r2 - 0.49Ï€r2)/Ï€r2 × 100
= 0.51 × 100
= 51
∴ Decrease in area = 51%
7. The area of a square is the same as the area of a circle. Their perimeters are in the ratio
A. 1 : 1
B. 2 : π
C. π : 2
D. √Ï€ : 2
Solution
Let the length of the side of the square be a
Let the radius if circle be r
Area of a square = a2
Area of circle = πr2
Area of a square = Area of a circle
a2 = πr2
⇒ a = √Ï€ × r
Perimeter of circle = 2Ï€r
Perimeter of square = 4a
= 4√Ï€r
Perimeter of square)/(Perimeter of circle) = 4√Ï€r/2Ï€r
Perimeter of circle = √Ï€/2
Ratio of perimeter of circle and square = √Ï€: 2
8. The circumference of a circle is equal to the sum of the circumferences of two circles having diameters 36 cm and 20 cm. The radius of the new circle is
A. 16 cm
B. 28 cm
C. 42 cm
D. 56 cm
Solution
Let the bigger circle be C1 and other circles be C2 and C3
Radius of circle C1 = r1
Diameter of circle C2 = 36 cm
Radius of circle C2 = r2 = 36/2 cm = 18 cm
Diameters of circle C3 = 20 cm
Radius of circle C3 = r3 = 20/2 cm = 10 cm
Circumference of circle C2 = 2Ï€r2
= 2 × Ï€ × 18 cm
= 36Ï€ cm
Circumference of circle C3 = 2Ï€r3
= 2 × Ï€ × 10 cm
= 20Ï€ cm
Circumference of circle C1 = Circumference of circle C2 + Circumference of circle C3
⇒ 2Ï€r1 = 2Ï€r2 + 2Ï€r3
⇒ 2Ï€r1 = 36Ï€ + 20Ï€
⇒ 2Ï€r1 = 56Ï€
⇒ r1 = 28 cm
Radius of circle C1 = r1 = 28 cm
9. The area of a circle is equal to the sum of the areas of two circles of radii 24 cm and 7 cm. The diameter of the new circle is
A. 25 cm
B. 31 cm
C. 50 cm
D. 62 cm
Solution
Let the bigger circle be C1 and other circles be C2 and C3
Radius of circles C1 = r1
Radius of circle C2 = r2 = 24 cm
Radius of circle C3 = r3 = 7 cm
r1 = 25 cm
Diameter of new circle = 25 × 2 cm
= 50 cm
10. If the perimeter of square is equal to the circumference of a circle then the ratio of their areas is
A. 4 : π
B. π: 4
C. π : 7
D. 7 : π
Solution
Let the length of the side of the square be a
Let the radius if circle be r
Perimeter of circle = 2Ï€r
Perimeter of square = 4a
Perimeter of circle = Perimeter of square
⇒ 2Ï€r = 4a
a = Ï€ × r/2
Area of square = a2
Area of circle = πr2
Area of square)/(Area of circle) = a2/Ï€r2
(Area of square)/(Area of circle) = (Ï€r/4)2/Ï€r2
(Area of square)(Area of circle) = π2r2/4πr2
Area of square)/Area of circle|) = π/4
Ratio of area of square to circle = π : 4
11. If the sum of the areas of two circles with radii R1 and R2 is equal to the area of a circle of radius R then
A. R1 + R2 = R
B. R1 + R2 < R
Let the three circles be C1, C2 and C
Area of circle C = Area of circle C1 + Area of circle C2
12. If the sum of the circumferences of two circles with radii R1 and R2 is equal to the circumference of a circle of radius R then
A. R1 + R2 = R
B. R1 + R2 > R
C. R1 + R2 < R
D. None of these
Solution
Let three circles be C1, C2 and C
Circumference of circle C = Circumference of circle C1 + Circumference of circle C2
⇒ 2Ï€R = 2Ï€R1 + 2Ï€R2
R = R1 + R2
13. If the circumference of a circle and the perimeter of a square are equal then
A. area of the circle = area of the square
B. (area of the circle) > (area of the square)
C. (area of the circle) < (area of the square)
D. None of these
Solution
Let the length of the side of the square be a
Let the radius if circle be r
Perimeter of circle = 2Ï€r
Perimeter of square = 4a
Perimeter of circle = Perimeter of square
2Ï€r = 4a
⇒ a = Ï€ × r/2
Area of square = a2
= (Ï€ × r/2)2
= Ï€/4 × Ï€r2
Area of circle = πr2
Seeing the co-efficient of πr2
1 > π/4
∴ Ï€r2 > Ï€/4 × Ï€r2
So, (area of the circle) > (area of the square)
14. The radii of two concentric circles are 19 cm and 16 cm respectively. The area of the ring enclosed by these circles is
A. 320 cm2
B. 330 cm2
C. 332 cm2
D. 340 cm2
Solution
Radius of circle 2 = r2 = 16 cm
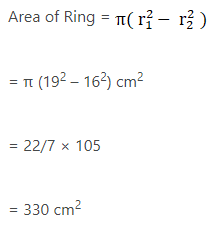
15. The areas of two concentric circles are 1386 cm2 and 962.5 cm2. The width of the ring is
A. 2.8 cm
B. 3.5 cm
C. 4.2 cm
D. 3.8 cm
Solution
Let the radius of circle 1 & 2 be R1 and R2 respectively
Area of circle 1 = 1386 cm2
R2 = 17.5 cm
Width of the ring = R1 – R2
= 21 – 17.5
= 3.5 cm
16. The circumferences of two circles are in the ratio 3 : 4. The ratio of their areas is
A. 3 : 4
B. 4 : 3
C. 9 : 16
D. 16 : 9
Solution
Circumference of circle C1 = 2Ï€r1
Circumference of circle C2 = 2Ï€r2
(Circumference of circle C1)/(Circumference of circle C2) = 2Ï€r1/2Ï€r2 = 3/4
r1/r2 = 3/4
∴ Ratio of two circles = 9 : 16
17. The areas of two circles are in the ratio 9 : 4. The ratio of their circumferences is
A. 3 : 2
B. 4 : 9
C. 2 : 3
D. 81 : 16
Solution
r1/r2 = 3/2
(Circumference of circle C1)/(Circumference of circle C2) = 2Ï€r1/2Ï€r2 = r1/r2 = 3/2
Ratio of their circumferences = 3 : 2
18. The radius of a wheel is 0.25 m. How many revolutions will it making in covering 11 km?
A. 2800
B. 4000
C. 5500
D. 7000
Solution
Radius of wheel = r = 0.25 m
Distance the wheel travels = 11 km = 11000 m
In 1 revolution wheel travels 2Ï€r distance
No. of revolutions a wheel makes = (distance travelled by the wheel)/2Ï€r
= 11000/(2Ï€ × 0.25)
= 11000/(2 × 22/7 × 0.25)
= (11000 × 7)/(2 × 22 × 0.25
= 7000 revolutions
19. The diameter of a wheel is 40 cm. How many revolutions will it make in covering 176 cm?
A. 140
B. 150
C. 160
D. 166
Solution
Diameter of wheel = 40 cm
Radius of wheel = r = 40/2 cm = 20 cm
Distance the wheel travels = 176 m = 17600 cm
In 1 revolution wheel travels 2Ï€r distance
No. of revolutions a wheel makes = (distance travelled by the wheel)/2Ï€r
= 17600/(2Ï€ × 20)
= 17600/(2 × 22/7 × 20)
= 17600 × 7)/(2 × 22 × 20)
= 140 revolutions
20. In making 1000 revolutions, a wheel covers 88 km. The diameter of the wheel is
A. 14 m
B. 24 m
C. 28 m
D. 40 m
Solution
Distance the wheels travels = 88 km = 88000 m
In 1 revolution wheel travels 2Ï€r distance
No. of revolutions a wheel makes = (distance travelled by the wheel)/2Ï€r
No. of revolutions a wheel makes = 1000
r = (distance travelled by the wheel)/(2Ï€ × No. of revolutions a wheel makes) = 88000/(2 × 22/7 × 1000)
= (88000 × 7)/(2 × 22 × 1000)
r = 14 m
Radius of wheel = 14 m
Diameter of wheel = 2 × 14 m = 28 m
21. The area of a sector of angle θ° of a circle with radius R is
A. 2πR2θ/180
B. 2πR2θ/360
C. πR2θ/180
D. πR2θ/360
Solution
Area of a sector of angle θ° of a circle with radius R = area of circle × Î¸/360
= πR2θ/360
22. The length of an arc of a sector of angle θ° of a circle with radius R is
A. 2πRθ /180
B. 2πRθ/360
C. πR2θ/180
D. πR2θ/360
Solution
Length of an arc of a sector of angle θ° of a circle with radius R
= Circumference of circle × Î¸/360
= 2πRθ/360
23. The length of the minute hand of a clock is 21 cm. The area swept by the minute hand in 10 minutes is
A. 231 cm2
B.210 cm2
C .126 cm2
D. 252 cm2
Solution
Length of the minute hand of a clock = 21 cm
∴ Radius = R = 21 cm
In 1 minute, minute hand sweeps 6°
So, in 10 minutes, minute hand will sweep 10 × 6° = 60°
Area swept by minute hand in 10 minutes = Area of a sector of angle of a circle with radius R = πR2θ/360
= 22/7 × 21 × 21 × 60/360
= 231 cm2
24. A chord of a circle of radius 10 cm subtends a right angle at the centre. The area of the minor segments (given, π = 3.14) is
A. 32.5 cm2
B. 34.5 cm2
C. 28.5 cm2
D. 30.5 cm2
Solution
Radius of circle = R = 10 cm
Area of minor segment = Area of sector subtending 90° - Area of triangle ABC
Area of sector subtending 90° = Ï€R2θ/ 360
= 3.14 × 10 × 10 × 90/360 cm2
= 78.5 cm2
Area of triangle ABC = 1/2 × AC × BC
= 1/2 × 10 × 10 cm2
= 50 cm2
Area of Minor segment = 78.5 cm2 – 50 cm2
= 28.5 cm2
25. In a circle of radius 21 cm, an arc subtends an angle of 60° at the centre. The length of the arc is
A. 21 cm
B. 22 cm
C. 18.16 cm
D. 23.5 cm
Solution
Radius of circle = R = 21 cm
Angle subtended by the arc = 60°
Length of an arc of a sector of angle θ° of a circle with radius R = 2Ï€Rθ/360
Length of arc = 2 × 22/7 × 21 × 60/360 cm
= 22 cm
26. In a circle of radius 14 cm, an arc subtends an angle of 120° at the centre. If √3 = 1.73 then the area of the segment of the circle is
A. 120.56 cm2
B. 124.63 cm2
C. 118.24 cm2
D. 130.57 cm2
Solution
Angle subtended by the arc = θ = 120°
Area of sector subtending 120° = Ï€R2θ/360
= 22/7 × 14 × 14 × 120/360 cm2
= 205.33 cm2
In triangle ABC
AC = BC = 14 cm = R
Area of triangle ABC = 1/2 × base × height
= 2 × 1/2 × R sin θ/2 × R × cos θ/2
= 2 × 1/2 × 14 × 14 × sin 60° × cos 60°
= 84.77 cm2
Area of segment = Area of sector subtending 120° - Area of triangle ABC
= 205.33 – 84.77 cm2
= 120.56 cm2
Areas of Circle, Sector & Segment
1. In the given figure, a square OABC has been inscribed in the quadrant OPBQ. If OA = 20 cm then the area of the shaded region is [take π = 3.14]
B. 228 cm2
C. 242 cm2
D. 248 cm2
Solution
Length of side of square = OA = 20 cm
Radius of Quadrant = Ï€R2 × Î¸/360 = 3.14 × 20√2 × 20√2 × 90/360
= 628 cm2
Area of square = a2 = 202 cm2 = 400 cm2
Area of Shaded region = Area of Quadrant – Area of Square
= 628 cm2 – 400 cm2
= 228 cm2
2. The diameter of a wheel is 84 cm. How many revolutions will it make to cover 792 m?
A. 200
B. 250
C. 300
D. 350
Solution
Diameter of wheel = 84 cm
Radius of wheel = r = 84/2 cm = 42 cm
Distance the wheel travels 792 m = 79200 cm
In 1 revolution wheel travels 2Ï€r distance
No. of revolutions a wheel makes = (distance travelled by the wheel)/2Ï€r
= 79200/(2Ï€ × 42)
= 79200/(2 × 22/7 × 42)
= (79200 × 7)/(2 × 22 × 42)
= 300 revolutions
3. The area of a sector of a circle with radius r, making an angle of x° at the centre is x
A. x/360 × 2Ï€r
B. x/180 × Ï€r2
C. x/360 × 2Ï€r
D. x/360 × Ï€r2
Solution
Area of a sector of angle θ° of a circle with radius R = area of circle = θ/360
= Ï€r2 × x°/360°
4. In the given figure, ABCD is a rectangle inscribed in a circle having length 8 cm and breadth 6 cm. If π = 3.14 then the area of the shaded region is
Solution
Given
Length of rectangle = 8 cm
Breadth of rectangle = 6 cm
Area of rectangle = length × breadth
= 8 × 6
= 48 cm2
Consider △ABC,
By Pythagoras theorem,
AC2 = AB2 + BC2
= 82 + 62
= 64 + 36
= 100
AC = √100 = 10 cm
⇒ Diameter of circle = 10 cm
Thus, radius of circle = 10/2 = 5 cm
Let the radius of circle be r = 5 cm
Then, Area of circle = πr2
= 22/7 × 5 × 5
= (22 × 25)/7
= 550/7
= 78.57 cm2
Area of shaded region = Area of circle – Area of rectangle
= 78.57 – 48
= 30.57 cm2
Hence, the area of shaded region is 30.57 cm2.
[None of the option is correct].
5. The circumference of a circle is 22 cm. Find its area. [Take π = 22/7]
Solution
Let the radius if circle be r
Circumference of circle = 22 cm
2Ï€r = 22 cm
⇒ 2 × 22/7 × r = 22 cm
⇒ r = 22 × 1/2 × 7/22 cm
⇒ r = 3.5 cm
Area of Circle = πr2
= 22/7 × 3.5 × 3.5 cm2
= 38.5 cm2
∴ Area of Circle = 38.5 cm2
6. In a circle of radius 21 cm, an arc subtends an angle of 60° at the centre. Find the length of the arc.
Solution
Radius of circle = R = 21 cm
Angle subtended by arc = 60°
Length of an arc of a sector of angle θ° of a circle with radius R
= Circumference of circle × Î¸/360
= 2πRθ/360
Length of arc = 2 × 22/7 × 21 × Î¸/360 cm
= 22 cm
Length of arc = 22 cm
7. The minute hand of a clock is 12 cm long. Find the area swept by it in 35 minutes.
Solution
Length of the minute hand of a clock = 12 cm
∴ Radius = R = 12 cm
In 1 minute, minute hand sweeps 6°
So, in 35 minutes, minute hand will sweep 35 × 6° = 210°
Area swept by minute hand in 35 minutes = Area of a sector of angle θ° of a circle with radius R = Ï€R2θ/360
= 22/7 × 12 × 12 × 60°/360°
= 264 cm2
Area swept by minute hand in 35 minutes = 264 cm2
8. The perimeter of a sector of a circle of radius 5.6 cm is 27.2 cm. Find the area of the sector.
Solution
Radius of circle = 5.6 cm
Perimeter of a sector of a circle = 2R + Circumference of circle × Î¸/360
= 2R + 2πRθ/360
Perimeter of a sector of a circle = 2 × 5.6 + 2 × 22/7 × 5.6 × Î¸/360 cm
= 27.2 cm
⇒ 2 × 22/7 × 5.6 × Î¸/360 = 27.2 – 11.2 cm
= 16 cm
⇒ θ = 16 × 1/2 × 1/5.6 × 7/22
⇒ θ = 163.63°
Area of sector = Ï€r2 × Î¸/360 = 22/7 × 5.6 × 5.6 × 163.63/360
= 44.8 cm2
∴ Area of sector = 44.8 cm2
9. A chord of a circle of radius 14 cm makes right angle at the centre. Find the area of the sector.
Solution
Radius of circle = R = 14 cm
Area of sector of circle of radius R = πR2θ/360
= 22/7 × 14 × 14 × 90/360 cm2
= 154 cm2
10. In the given figure, the sectors of two concentric circles of radii 7 cm and 3.5 cm are shown. Find the area of the shaded region.
Given,
Radius of smaller circle = R1 = 3.5 cm
Radius of bigger circle = R2 = 7 cm
Angle subtended = 30°
11. A wire when bent in the form of an equilateral triangle encloses an area of 121√3 cm2. If the same wire is bent into form of a circle, what will be the area of the circle? [Take Ï€ = 22/7]
Solution
Let the sides of equilateral triangle be a cm
Area of equilateral triangle = 121√3 cm2
Area of equilateral triangle = √3/4 × a2
⇒ √3/4a2 = 121√3
⇒ a2 = 121√3 × 4√3 = 121 × 4 cm2
⇒ a2 = 484 cm2
⇒ a = 22 cm
Perimeter of equilateral triangle = 3a
= 3 × 22 cm
= 66 cm
Perimeter of equilateral triangle = Circumference of circle
Circumference of circle = 66 cm
Let the radius of circle be r
Circumference of circle = 2Ï€r
⇒ 2Ï€r = 66 cm
⇒ 2 × 22/7 × r = 66 cm
⇒ r = 66 × 1/2 × 7/22 cm
⇒ r = 10.5 cm
Area of circle = Ï€r2 = 22/7 × 22/7 × 10.5 × 10.5 cm2
= 346.5 cm2
12. The wheel of a cart is making 5 revolutions per second. If the diameter of the wheel is 84 cm, find its speed in km per hour. [Take π = 22/7]
Solution
Diameter of the wheel = 84 cm
Let the radius of the wheel be R cm
Radius of the wheel = 84/2 cm = 42 cm
No. of revolutions wheel makes = 5 rev/sec
Since, 1 revolution = 2Ï€R
Speed of the wheel = 5 × 2Ï€R rev/sec
= 5 × 2 × 22/7 × 42
= 1320 cm/sec
= 13.20 m/sec
= 13.20 × 3600/1000 km/h = 47.52 km/h
Since, 1 m/sec = 3600/1000 km/h
13. OACB is a quadrant of a circle with centre O and its radius is 3.5 cm. If OD = 2 cm, find the area of (i) the quadrant OACB
(ii) the shaded region. [Take π = 22/7]
Radius of circle = R = 3.5 cm
OD = 2 cm
OA = OB = R = 3.5 cm
Since, OACB is a quadrant of a circle
∴ Angle subtended by it at the centre = 90°
(i) Area of quadrant = πR2θ/360
= 22/7 × 3.5 × 3.5 × 90°/360° cm2
= 9.625 cm2
(ii) Area of shaded region = Area of quadrant – Area of triangle OAD
Area of triangle OAD = 1/2 × base × height
= 1/2 × OA × OD
= 1/2 × 3.5 × 2 cm2
= 3.5 cm2
Area of shaded region = 9.625 cm2 – 3.5 cm2
= 6.125 cm2
14. In the given figure, ABCD is a square each of whose side measures 28 cm. Find the area of the shaded region. [Take π = 22/7]
Length of the sides of square = 28 cm
Area of square = a2 = 282 cm2
= 784 cm2
Since, all the circles are identical so, they have same radius
Let the radius of circle be R cm
From the figure 2R = 28 cm
From the figure 2R = 28 cm
R = 28/2 cm
⇒ R = 14 cm
Quadrant of a circle subtends 90° at the centre.
Area of quadrant of circle = πR2θ/360
= 22/7 × 14 × 14 × 90°/360° cm2
= 154 cm2
Area of 4 quadrants of circle = 154 × 4 cm2
= 616 cm2
Area of shaded region = Area of square – Area of 4 quadrants of circle
= 784 cm2 – 616 cm2
= 168 cm2
15. In the given figure, an equilateral triangle has been inscribed in a circle of radius 4 cm. Find the area of the shaded region. [Take Ï€ = 3.14 and √3 = 1.73]
OD perpendicular to AB is drawn
△ABC is equilateral triangle,
∠A = ∠B = ∠C = 60°
∠OAD = 30°
OD/AO = sin 30°
AO = 4 cm
OD/AO = 1/2
⇒ OD = 1/2 × 4 cm
⇒ OD = 2 cm
AD2 = OA2 – OD2
= 42 – 22
= 16 – 4
= 12 cm2
AD = 2√3 cm
AB = 2 × AD
= 2 × 2√3 cm
= 4√3 cm
Area of triangle ABC = √3/4 × AB2
= √3/4 × 4√3 × 4√3
= 20.71 cm2
Area of circle = πR2
= 3.14 × 4 × 4 cm2
= 50.24 cm2
Area of shaded region = 29.53 cm2
16. The minute hand of a clock is 7.5 cm long. Find the area of the face of the clock described by the minute hand in 56 minutes.
Solution
Length of minute hand = 7.5 cm
In a clock, length of minute hand = radius
Radius = R = 7.5 cm
In 1 minute, minute hand moves 6°
So, in 56 minutes, minute hand moves 56 × 6° = 360°
Area described by minute hand = Ï€R2θ/360°
= 22/7 × 7.5 × 7.5 × 336°/360° cm2
= 165 cm2
17. A racetrack is in the form of a ring whose inner circumference is 352 m and outer circumference is 396 m. Find the width and the area of the track.
Solution
Let the inner radius be R1 and outer radius be R2
Inner circumference = 2Ï€R1 = 352 m
⇒ 2 × 22/7 × R1 = 3652 m
⇒ R1 = 352 × 1/2 × 7/22
⇒ R1 = 56 m
Outer Circumference = 2Ï€R2 = 396 m
⇒ 2 × 22/7 × R2 = 396 m
⇒ R2 = 396 × 1/2 × 7/22 m
⇒ R2 = 63 m
Width of the track = R2 – R1 = 63 m – 56m = 7 m
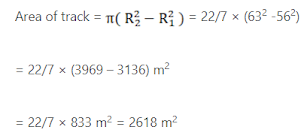
Solution
Chord AB subtends an angle of 60° at the centre
Radius = 30 cm
Let radius be R
In triangle ABC, AC = BC
So, ∠CAB = ∠CBA
∠ACB + CAB + ∠CBA = 180°
⇒ 60° + 2∠CAB = 180°
⇒ 2∠CAB = 180° – 60°= 120°
⇒ ∠CAB = 120°/2 = 60°
⇒ ∠CAB = ∠CBA = 60°
∴ △ABC is a equilateral triangle
Length of side of an equilateral triangle = radius of circle = 30 cm
Area of equilateral triangle = √3/4 × side2
= 1.732 × 30 × 30 cm2
= 389.7 cm2
Area of sector ACB = πR2θ/360
= 3.14 × 30 × 30 × 60°
= 471.45 cm2
Area of minor segment = Area of sector ACB – Area of △ABC
= 471.45 cm2 – 389.7 cm2
= 81.75 cm2
Area of circle = Ï€R2 = 3.14 × 30 × 30 cm2
= 2828.57 cm2
Area of major segment = Area of circle – Area of minor segment
= 2826 cm2 – 81.75 cm2
= 2744.25 cm2
19. Four cows are tethered at the four corners of a square field of side 50 m such that each can graze the maximum unshared area. What area will be left ungrazed? [Take π = 3.14]
Solution
Length of side of square = 50 m
Length of side of square = 2 × Radius of quadrant
Radius of quadrant = R = 50/2 m = 25 m
Area of square = side2
= 502 m2
= 2500 m2
Area of quadrant = 1/4Ï€R2
= 1/4 × 3.14 × 25 × 25 m2
= 490.625 m2
Area of 4 quadrants = 4 × 490.625 m2
= 1962.5 m2
Area left ungrazed = Area of shaded part
= Area of square – Area of 4 quadrants
= 2500 m2 – 1962.5 m2
= 537.5 m2
20. A square tank has an area of 1600 m2. There are four semi-circular plots around it. Find the cost of turfing the plots at Rs 12.50 per m2. [Take π = 3.14]
Solution
Let the length of side of the square tank be a
Area of square tank = a2 = 1600 m2
⇒ a = √1600 m
= 40 m
Let the radius of semicircle be R
From the figure we can see that
Length of the side of the square = Diameter of semicircle
40 m = 2 × R
⇒ R = 40/2 m
⇒ R = 20 m
Area of semi-circle = 1/2 πR2
= 1/2 × 3.14 × 20 × 20 m2
= 628 m2
Area of 4 semi-circles = 4 × 628 m2
= 2512 m2
Cost of turfing the plots = Rs 12.50 per m2
Cost of turfing = Cost of turfing per m2 × Area of 4 semicircle
= Rs 12.50 × 2512
= Rs 31400