RD Sharma Solutions for Class 10 Chapter 7 Statistics Exercise 7.6

RD Sharma Solutions Chapter 7 Statistics Exercise 7.6 Class 10 Maths

Chapter Name	RD Sharma Chapter 7 Statistics
Book Name	RD Sharma Mathematics for Class 10
Other Exercises	Exercise 7.1 Exercise 7.2 Exercise 7.3 Exercise 7.4 Exercise 7.5
Related Study	NCERT Solutions for Class 10 Maths

Exercise 7.6 Solutions

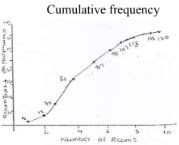
1. Draw an given by less than method for the following data:

No. of rooms:	1	2	3	4	5	6	7	8	9	10
No. of houses:	4	9	22	28	24	12	8	6	5	2

Solution
We first prepare the cumulative frequency distribution table by less than method as given be :

Now, we mark the upper class limits along x - axis and cumulative frequency along y - axis. 
Thus we plot the point (1,4), (2, 3), (3, 35), (4, 63), (5, 87), (6, 99), (7,107), (8, 113), (9, 118), (10, 120).

2. The marks scored by 750 students in an examination are given in the form of a frequency distribution table:

Marks	No. of students	Marks	No. of students
600 – 640	16	760 – 800	172
640 – 680	45	800 – 840	59
680 – 720	156	840 – 800	18
720 – 760	284

Prepare a cumulative frequency table by less than method and draw an ogive.

Solution

We first prepare the cumulative frequency table by less than method as given below :

Now, we mark the upper class limits along x-axis and cumulative frequency along y-axis on a suitable gear.
Thus, we plot the points (640, 16) (680, 61), (720, 217), (760, 501), (600, 673), (840, 732) and (880, 750).

3. Draw an ogive to represent the following frequency distribution:

Class – interval :	0 – 4	5 – 9	10 – 14	15 – 19	20 – 24
No. of students:	2	6	12	5	3

Solution

The given frequency of distribution is not continuous so we first make it continuous and prepare the cumulative frequency distribution as under

Now, we mark the upper class limits along x-axis and cumulative frequency along y-axis.

Thus we plot the points (4, 5, 2), (9, 5, 8), (14, 5, 08), (19, 5, 23) and (24, 5, 26)
Cumulative frequency.

4. The monthly profits (in Rs.) of 100 shops are distributed as follows:

Profits per shop:	0 – 50	50 – 100	100 – 150	150 – 200	200 – 250	250 – 300
No. of shops:	12	18	27	20	17	6

Draw the frequency polygon for it.

Solution

We have, 

5. The following table gives the height of trees:

Height	No. of trees
Less than 7	26
Less than 14	57
Less than 21	92
Less than 28	134
Less than 35	216
Less than 42	287
Less than 49	341
Less than 56	360

Draw ‘less than’ ogive and ‘more than’ ogive.

Solution

Less than method, 
It is given that, 

Now, we mark the upper class limits along x - axis and cumulative frequency along y - axis.
Thus we plot the points (7, 26) (14, 57) (21, 92) (28, 134) (35, 216) (42, 287) (49, 341) (56, 360)
More than method: we prepare the cf table by more than method as given below:
Now, we mark on x - axis lower class limits, y - axis cumulative frequency 
Thus, we plot graph at(0,360)(7, 334)(14,303)(21, 268)(28,226)(35,144)(42, 73)(49, 19)

6. The annual profits earned by 30 shops of a shopping complex in a locality give rise to the following distribution:

Profit (in lakhs in Rs)	Number of shops(frequency)
More than or equal to 5	30
More than or equal to 10	28
More than or equal to 15	16
More than or equal to 20	14
More than or equal to 25	10
More than or equal to 30	7
More than or equal to 35	3

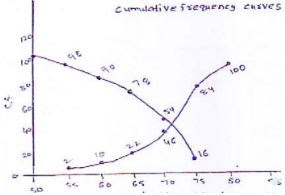
Draw both ogives for the above data and hence obtain the median.

Solution

More than method 

Now, we mark on x - axis lower class limits, y - axis cumulative frequency 
Thus, we plot the points. (5, 30)(10, 28)(15, 16)(20, 14)(25, 10)(30, 7) and (35, 3)
Less than method. 

Now, we mark the upper class limits along x - axis and cumulative frequency along y - axis.
Thus we plot the points, (10, 2) (15, 14)(20, 16)(25, 20)(30, 23)(35, 27)(40, 30)
We find that the two types of curves intersect of point P from point L it is drawn on x - axis. 
The value of a profit corresponding to M is  17.5 lakh, Hence median is 17.5 lakh

7. The following distribution gives the daily income of 50 workers of a factory:

Daily income (in Rs):	100 – 120	120 – 140	140 – 160	160 – 180	180 – 200
Number of workers:	12	14	8	6	10

Convert the above distribution to a less than type cumula five frequency distribution and draw its ogive.

Solution

We first prepare the cumulative frequency table by less than method as given below.

8. The following table gives production yield per hectare of wheat of 100 farms of a village:

Production yield in kg per hectare:	50 – 55	55 – 60	60 – 65	65 – 70	70 – 75	75 – 80
Number of farms:	2	8	12	24	38	16

Draw ‘less than’ ogive and ‘more than’ ogive.

Solution

Less than method: 
Cumulative frequency table by less than method. 

Now, we mark on x - axis upper class limit, y - axis cumulative frequencies. 
We plot the points(50, 100)(55, 98)(60, 90)(65, 78)(70, 54)(75, 16)

9. During the medical check – up of 35 students of a class, their weights were recorded as follows:

Weight (in kg)	No. of students
Less than 38	0
Less than 40	3
Less than 42	5
Less than 44	9
Less than 46	14
Less than 48	28
Less than 50	32
Less than 52	35

Draw a less than type ogive for the given data. Hence, obtain the median weight from the graph and verify the result by using the formula.

Solution

Less than method 
It is given that 
On x - axis upper class limits. Y - axis cf. 
We plot the points (38, 0)(40, 3)(42, 5)(44, 9)(46, 4)(48, 28)(50, 32)(52, 35) 
More than method: Cf table 

x - axis lower class limits on y - axis - cf 
We plot the points(38, 35)(40, 32)(42, 30)(44, 26)(46, 21)(48, 7)(50, 3)

We find the two types of curves intersect at a point P. From point P, from P perpendicular PM is draw on x - axis. 
The verification, 
We have