RD Sharma Solutions for Class 10 Chapter 7 Statistics Exercise 7.5

RD Sharma Solutions Chapter 7 Statistics Exercise 7.5 Class 10 Maths

Chapter Name	RD Sharma Chapter 7 Statistics
Book Name	RD Sharma Mathematics for Class 10
Other Exercises	Exercise 7.1 Exercise 7.2 Exercise 7.3 Exercise 7.4 Exercise 7.6
Related Study	NCERT Solutions for Class 10 Maths

Exercise 7.5 Solutions

1. Find the mode of the following data:
(i) 3,5,7,4,5,3,5,6,8,9,5,3,5,3,6,9,7,4
(ii) 3,3,7,4,5,3,5,6,8,9,5,3,5,3,6,9,7,4
(iii) 15,8,26,25,24,15,18,20,24,15,19,15

Solution

2. The shirt sizes worn by a group of 200 persons, who bought the shirt from a store, are as follows:

Shirt size:	37	38	39	40	41	42	43	44
Number of persons:	15	25	39	41	36	17	15	12

Find the model shirt size worn by the group.

Solution

Shirt size:	37	38	39	40	41	42	43	44
Frequency (f)	15	25	39	41	36	17	15	12

Model shirt size = 40 because it occurs maximum number of times

3. Find the mode of the following distribution.

(i)

Class interval:	0 – 10	10 – 20	20 – 30	30 – 40	40 – 50	50 – 60	60 – 70	70 – 80
Frequency:	5	8	7	12	28	20	10	10

(ii)

Class – inteval:	10 – 15	15 – 20	20 – 25	25 – 30	30 – 35	35 – 40
Frequency:	30	45	75	35	25	15

(iii)

Class – interval:	25 – 30	30 – 35	35 – 40	40 – 45	45 – 50	50 – 60
Frequency:	25	34	50	42	38	14

Solution

4. Compare the modal ages of two groups of students appearing for an entrance test:

Age(in years):	16 – 18	18 – 20	20 – 22	22 – 24	24 – 26
Group A	50	78	46	28	23
Group B	54	89	40	25	17

Solution

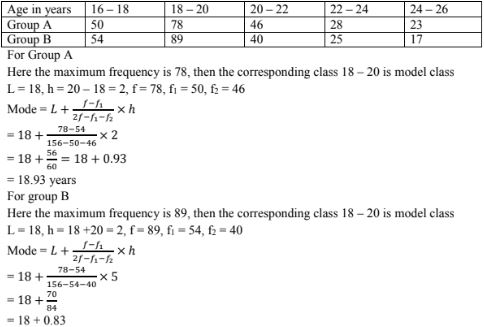
= 18.83
Hence the mode of age for the group A is higher than group B.

5. The marks in science of 80 students of class X are given below: Find the mode of the marks obtained by the students in science.

Marks:	0 – 10	10 – 20	20 – 30	30 – 40	40 – 50	50 – 60	60 – 70	70 – 80	80 – 90	90 – 100
Frequency:	3	5	16	12	13	20	5	4	1	1

Solution

6. The following is the distribution of height of students of a certain class in a certain city:

Height(in cm):	160 – 162	163 – 165	166 – 168	169 – 171	172 – 174
No. of students:	15	118	142	127	18

Find the average height of maximum number of students.

Solution

7. The following table shows the ages of the patients admitted in a hospital during a year:

Age(in years):	5 – 15	15 – 25	25 – 35	35 – 45	45 – 55	55- 65
No. of students:	6	11	21	23	14	5

Find the mode and the mean of the data given above. Compare and interpret the two measures of central tendency.

Solution

8. The following data gives the information on the observed lifetimes (in hours) of 225 electrical components:

Lifetimes (in hours):	0 – 20	20 – 40	40 – 60	60 – 80	80 – 100	100 – 120
No. of components:	10	35	52	61	38	29

Determine the modal lifetimes of the components.

Solution
From data as given above we may observe that maximum class frequency 61 belonging to 
class interval 60 - 80. 
So, modal class 60 - 80 

9. The following data gives the distribution of total monthly houshold expenditure of 200 families of a village. Find the modal monthly expenditure of the families.Also, find the mean monthly expenditure:

Expenditure (in Rs.)	Frequency	Expenditure(in Rs.)	Frequency
1000 – 1500	24	3000 – 3500	30
1500 – 2000	40	3500 – 4000	22
2000 – 2500	33	4000 – 4500	16
2500 – 3000	28	4500 – 5000	7

Solution

10. The following distribution gives the state – wise teacher – student ratio in higher secondary schools of India. Find the mode and mean of this data. Interpret, the two measures:

Number of students per teacher	Number of states/U.T.	Number of students per teacher	Number of states/U.T.
15 – 20	3	35 – 40	3
20 – 25	8	40 – 45	0
25 – 30	9	45 – 50	0
30 – 35	10	50 – 55	2

Solution

11. The given distribution shows the number of runs scored by some top batsmen of the world in one – day international cricket matches.

Runs scored	No. of batsman	Runs scored	No. of batsman
3000 – 4000	4	7000 – 8000	6
4000 – 5000	18	8000 – 9000	3
5000 – 6000	9	9000 – 10000	1
6000 – 7000	7	10000 – 11000	1

Find the mode of the data.

Solution

From the given data we may observe that maximum class frequently is 18 belonging to 
class interval  4000 - 5000
So modal class 4000 - 5000 
Lower limit(1) of model class = 4000

12. A student noted the number of cars passing through a spot on a road for loo periods each of 3 minutes and summarized it in the table given below. Find the mode of the data:

Solution

From the given data we may observe that maximum class internal frequency is 200 belonging to modal class = 40 - 50 

13. The following frequency distribution gives the monthly consumption of electricity of 68 consumers of a locality. Find the median, mean and mode of the data the compare them.

Monthly consumption(in units)	65 – 85	85 – 105	105 – 125	125 – 145	145 – 165	165 – 185	185 – 205
No. of consumers:	4	5	13	20	14	8	4

Solution

14. 100 surnames were randomly picked up from a local telepone directly and the frequency distribution of the number of letters in the English alphabets in the surnames was obtained as follows:

Number of letters:	1 – 4	4 – 7	7 – 10	10 – 13	13 – 16	16 – 19
Number surnames:	6	30	40	16	4	4

Determine the median number of letters in the surnames. Find the mean number of letters in the surnames. Also, find the modal size of the surnames.

Solution

15. Find the mean, median and mode of the following data:

Classes:	0 – 20	20 – 40	40 – 60	60 – 80	80 – 100	100 – 120	120 – 140
Frequency:	6	8	10	12	6	5	3

Solution

16. Find the mean, median and mode of the following data:

Classes :	0 – 50	50 – 100	100 – 150	150 – 200	200 – 250	250 – 300	300 – 350
Frequency:	2	3	5	6	5	3	1

Solution

17. The following table gives the daily income of 50 workers of a factory:

Daily income(in Rs)	100 – 120	120 – 140	140 – 160	160 – 180	180 – 200
Number of workers:	12	14	8	6	10

Find the mean, mode and median of the above data.

Solution