RD Sharma Solutions for Class 10 Chapter 5 Trigonometric Ratios Exercise 5.1

RD Sharma Solutions Chapter 5 Trigonometric Ratios Exercise 5.1 Class 10 Maths

Chapter Name	RD Sharma Chapter 5 Trigonometric Ratios
Book Name	RD Sharma Mathematics for Class 10
Other Exercises	Exercise 5.2 Exercise 5.3
Related Study	NCERT Solutions for Class 10 Maths

Exercise 5.1 Solutions 

1. In each of the following one of the six trigonometric ratios is given. Find the values of the other trigonometric ratios. 

Solution

2. In a ΔABC, right angled at B, AB = 24 cm, BC = 7cm. Determine 
(i) SinA, CosA
(ii) SinC, CosC

Solution

ΔABCis right angled at B 
AB = 24cm, BC = 7cm. 

3. In Fig below, Find tan P and cot R. Is tan P = cot R?

Solution

4. If sin A = 9/41,  compute cosA and tanA 

Solution

5.Given 15 cotA = 8, find Sin A and secA. 

Solution

15 cot A = 8, find Sin A and sec A 

6. In ΔPQR, right angled at Q, PQ = 4 cm and RQ = 3 cm. Find the values of sin P, sin R, sec P and sec R.

Solution

ΔPQR, right angled at Q.

7. If cot θ =7/8, evaluate : 

(i) [(1 + sinθ)(1 - sinθ)]/[(1 + cosθ)(1 - cosθ)
(ii) Cot²θ 

Solution

8. If 3cotA = 4, check whether (1 - tan²A)/(1 + tan²A) = cos²A - sin²A or not. 

Solution

9. If tan θ = a/b, find the value of (cosθ + sinθ)/(cosθ - sinθ).

Solution

10. If 3 tanθ = 4, find the value of (4cosθ - sinθ)/(2cosθ + sinθ).

Solution

11. If 3cotθ = 2, find the value of = (4sinθ - 3cosθ)/(2sinθ + 6sinθ)

Solution

12. If tanθ = a/b, prove that (a sinθ - b cosθ)/(a sinθ + b cosθ) = (a² - b²)/(a² + b²). 

Solution

13. If secθ = 13/5, show that (2cosθ - 3cosθ)/(4sinθ - 9cosθ) = 3 

Solution

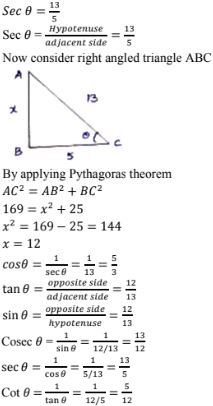
14. If cosθ = 12/13, show that sinθ (1 - tanθ) = 35/156 . 

Solution

15. If cotθ = 1/√3, show that  (1 - cos²θ)/(2 - sin²θ) = 3/5 . 

Solution

16. If tanθ = 1/√7 
(cosec²θ  - sec²θ)/(cosec²θ + sec²θ) = 3/4

Solution

17. If Sinθ = 12/13 find (sin²θ - cos²θ)/(2 sinθ cosθ) × 1/tan²θ 

Solution

18.  If secθ = 5/4 , find the value of (sinθ - 2cosθ)/(tanθ - cotθ) 

Solution

19. Tanθ = 12/13 
Find (2sinθ cosθ)/(cos²θ - sin²θ)

Solution

21. If cosθ = 3/5, find the value of  (sinθ - 1/tanθ)/2tanθ . 

Solution

22. If sinθ = 3/5, evaluate  (cosθ - 1/tanθ)/2cotθ

Solution

23. If sec A = 5/4, verify that (3sinA - 4sin³A)/(4cos³A - 3cos A) = (3tanA - tan³A)/(1 - 3tan²A).

Solution

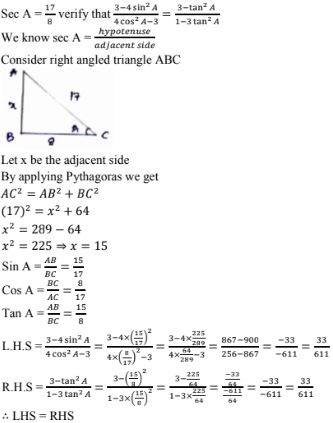
24. If sec A = 17/8, verify that (3 - 4sin²A)/(4cos²A - 3) = (3 - tan²A)/(1 - 3tan²A) 

Solution

25. If cot θ = 3/4, prove that √(secθ - cosecθ)/(secθ + cosecθ) = 1/√7

Solution

27. If tanθθ = 24/7, find that sin θ + cos θ

Solution

28. If sin θ = a/b, find sec θ + tanθ in terms of a and b. 

Solution

29.  If 8 tan A = 15, find  sinA - cosA.

Solution

30. If 3cosθ - 4sinθ = 2cosθ + sinθ Find tanθ . 

Solution

3cosθ - 2cosθ = 4sinθ + sinθ find tanθ
3cosθ - 2cosθ = sinθ + 4sinθ
cosθ = 5sinθ
Dividing both side by use we get 
cosθ/cosθ = 5sinθ/cosθ 
1 = 5tanθ
⇒ tanθ = 1 

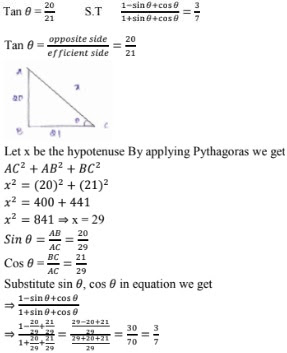
31.  If tanθ = 20/21, show that (1- sinθ + cosθ)/(1 + sinθ + cosθ) = 3/7

Solution

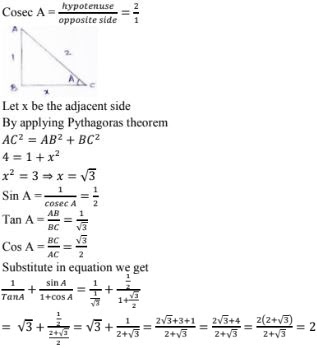
32. If Cosec A = 2 find 1/tanA + sinA/(1 + cosA)

Solution

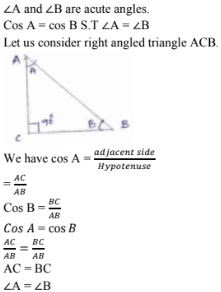
33. If ∠A and ∠B are acute angles such that cos A = cos B, then show that ∠A = ∠B. 

Solution

34. If ∠A and ∠P are acute angles such that tan A = tan P, then show that ∠A = ∠P. 

Solution

A and P are acute angle tan A = tan P
S.T.     ∠A = ∠P
Let us consider right angled triangle ACP, 

35. In a ΔABC, right angled at A, if tan C = √3, find the value of sin B cos C + cos B sin C .

Solution

In a Δle  ABC right angled at A tan C = √3 
Find sin B cos C + cos B sin C 

36. State whether the following are true or false. Justify your answer. 
(i) The value of tan A is always less than I. 
(ii)  Sec A = 12/5 for some value of angle A. 
(iii) Cos A is the abbreviation used for the cosecant of angle A. 
(iv) Sin θ = 4/3 for some angle θ. 

Solution

(a) Tan A ∠1 
Value of tan A at 45° i.e., tan 45 = 1 
As value of A increases to 90° 
Tan A becomes infinite 
So given statement is false. 

(b) Sec A = 12/5 for some value of angle of 
M - I
Sec A = 2.4 
Sec A > 1 
So given statement is True 
M - II
For sec A = 12/5 
For sec A = 12/5 we get adjacent side = 13 

We get a right angle Δle 
Subtending 9i at B. 
So, given statement is true. 

(c)  Cos A is the abbreviation used for cosecant of angle A. 

The given statement is false 
∴ Cos A is abbreviation used for cos of angle A but not for cosecant of angle A. 

(d) Cot A is the product of cot A and A 
Given statement is false 
∵ cot A is co - tangent of angle A and co - tangent of angle A = (adjacent side)/(opposite side) 
(e)  Sin θ = 4/3  for some angle θ 
Given statement is false 
Since value of sin θ is less than (or) equal to one. Here value of sin θ exceeds one, so given statement is false.