RD Sharma Solutions Chapter 3 Pair of Linear Equation in Two Variables Exercise 3.3 Class 10 Maths

Chapter Name | RD Sharma Chapter 3 Pair of Linear Equation in Two Variables |
Book Name | RD Sharma Mathematics for Class 10 |
Other Exercises |
|
Related Study | NCERT Solutions for Class 10 Maths |
Exercise 3.3 Solutions
Solve the following systems of equations:
1. 11x + 15y + 23 = 0
7x – 2y – 20 = 0
Solution
The given system of equation is
11x + 15y + 23 = 0 …(i)
7x – 2y – 20 = 0 …(ii)
From (ii), we get
2y = 7x – 20
⇒ y = (7x – 20)/2
Substituting y = (7x – 20)/2 in (i) we get
11x + 15[(7x – 20)/2] + 23 = 0
⇒ 11x + (105x – 300)/2 + 23 = 0
⇒ (22x + 105x – 300 + 46)/2 = 0
⇒ 127x – 254 = 0
⇒ 127x = 254
⇒ x = 254/127 = 2
Putting x = 2 in y = (7x – 20)/2 we get
⇒ y = (7×2 – 20)/2
= (14 – 20)/2
= -6/2 = -3
Hence, the solution of the given system of equation is x = 2, y = -3.
2. 3x – 7y + 10 = 0
y – 2x – 3 = 0
Solution
The given system of equation is
3x – 7y + 10 = 0 …(i)
y – 2x – 3= 0 …(ii)
From (ii), we get
y = 2x + 3
Substituting y = 2x + 3 in (i) we get
3x – 7(2x + 3) + 10 = 0
⇒ 3x + 14x – 21 + 10 = 0
⇒ - 11x = 11
⇒ x = 11/-11 = -1
Putting x = - 1 in y = 2x + 3, we get
⇒ y = 2×(-1) + 3
= -2 + 3
= 1
⇒ y = 1
Hence, the solution of the given system of equations is x = -1, y = 1.
3. 0.4x + 0.3x = 1.7
0.7x + 0.2y = 0.8
Solution
The given system of equation is
0.4x + 0.3y = 1.7 …(i)
0.7x - 0.2y = 0.8 …(ii)
Multiplying both sides of (i) and (ii), by 10, we get
4x + 3y = 17 …..(iii)
7x – 2y = 8 ….(iv)
From (iv), we get
7x = 8 + 2y
⇒ x = (8 + 2y)/7
Substituting x = (8 +2y)/7 in (iii), we get
4[(8+2y)/7] + 3y = 17
⇒ (32 + 8y)7 + 3y = 17
⇒ 32 + 29y = 17×7
⇒ 29y = 87
⇒ y = 87/29 = 3
Putting y = 3 in x = (8+2y)/7, we get
x = (8 + 2×3)/7
= (8 + 6)/7
= 14/7 = 2
Hence, the solution of the given system of equation is x = 2, y = 3.
4. x/2 +y = 0.8
Solution
x/2 + y = 0.8
And 7/(x+y/2) = 10
∴ x + 2y = 1.6 and (7×2)/(2x + y) = 10
x + 2y = 1.6 and 7 = 10x + 5y
Multiply first equation by 10
10x + 20y = 16 and 10x + 5y = 7
Subtracting the two equations
15y = 9
y = 9/15 = 3/5
x = 1.6 – 2(3/5) = 1.6 – 6/5 = 2/5
Solution is (2/5, 3/5)
5. 7(y+3 ) – 2(x + 3) = 14
4(y – 2) + 3(x – 3) = 2
Solution
The given system of equation is
7(y+3 ) – 2(x + 3) = 14 …(i)
4(y – 2) + 3(x – 3) = 2 …(ii)
From (i), we get
7x + 21 – 2x – 4 = 14
⇒ 7y = 14 + 4 – 21 + 2x
⇒ y = (2x – 3)/7
From (ii), we get
4y – 8 + 3x – 9 = 2
⇒ 4y + 3x – 17 – 2 = 0
⇒ 4y + 3x – 19 = 0 …(iii)
Substituting y = (2x – 3)/7 in (iii), we get
4[(2x – 3)/7] + 3x – 19 = 0
⇒ (8x – 12)/7 + 3x – 19 = 0
⇒ 8x – 12 + 21x – 133 = 0
⇒ 29x – 145 = 0
⇒ 29x = 145
⇒ x = 145/29 = 5
Putting x = 5 in y = (2x – 3)/7, we get
y = (2×5 – 3)/ 7
= 7/7 = 1
⇒ y = 1
Hence, the solution of the given system of equations is x = 5, y = 1.
6. x/7 + y/3 = 5
x/2 – y/9 = 6
Sol: The given system of equation is
x/7 + y/3 = 5 …(i)
x/2 – y/9 = 6 …(ii)
From (i), we get
(3x +7y)/21 = 5
⇒ 3x + 7y = 105
⇒ 3x = 105 – 7y
⇒ x = (105 – 7y)/3
From (ii), we get
(9x – 2y)/18 = 6
⇒ 9x – 2y = 108 ...(iii)
Substituting x = (105 – 7y)/3 in (iii), we get
9[(105 – 7y)/3] – 2y = 108
⇒ (948 – 63y)/3 – 2y = 108
⇒ 945 – 63y – 6y = 108×3
⇒ 945 – 69y = 324
⇒ 945 – 324 = 69y
⇒ 69y = 621
⇒ y = 621/69 = 9
Putting y = 9 in x = (1105 – 7y)/3, we get
x = (105 – 7×9)/3 = (105 – 63)/3
⇒ x = 42/3 = 14
Hence, the solution of the given system of equations is x = 14, y = 9.
7. x/3 + y/4 = 11
5x/6 – y/3 = 7
Solution
The given system of equations is
x/3 + y/4 = 11 …(i)
5x/6 – y/3 = 7 …(ii)
From (i) , we get
(4x + 3y)/12 = 11
⇒ 4x + 3y = 132 …(iii)
From (ii), we get
(5x + 2y)/6 = -7
⇒ 5x – 2y = - 42 …(iv)
Let us eliminate y from the given equations. The coefficients of y in the equations (iii) and (iv) are 3 and 2 respectively. The L.C.M of 3 and 2 is 6. So, we make the coefficient of y equal to 6 in the two equations.
Multiplying (iii) by 2 and (iv) by 3, we get
8x + 6y = 264 …(v)
15x – 6x = -126 …(vi)
Adding (v) and (vi), we get
8x + 15x = 264 – 126
⇒ 23x = 138
⇒ x = 138/23 = 6
Substituting x = 6 in (iii) , we get
4 ×6 + 3y = 132
⇒ 3y = 132 – 24
⇒ 3y = 108
⇒ y = 108/3 = 36
Hence, the solution of the given system of equations is x = 6, y= 36.
8. 4u + 3y = 8
6u – 4y = -5
Solution
Taking 1/x = u, then given equations become
4u + 3y =8 ...(i)
6u – 4y = - 5 …(ii)
From (i) we get
4u = 8 – 3y
⇒ u = (8 – 3y)/4
Substitutig u = (8 – 3y) /4 in (ii), we get
From (ii), we get
6[(8 – 3y)/4] – 4y = -5
⇒ 3(8 – 3y)/2 – 4y = - 5
⇒ (24 – 9y)/2 – 4y = - 5
⇒ (24 – 9y – 8y)/2 = -5
⇒ 24 – 17y = -10
⇒ -17y = -10 – 24
⇒ -17y = -34
⇒ y = -34/-17 = 2
Putting y = 2, in u = (8 – 3y)/4, we get
u = (8 – 3×2)/4 = (8-6)/4= 2/4= ½
Hence, x = 1/u = 2
So, the solution of the given system of equation is x = 2, y = 2.
9. x + y/2 = 4
x/3 + 2y = 5
Solution
The given system of equation is
x + y/2 = 4 …(i)
x/3 + 2y = 5 …(ii)
From (i) , we get
(2x + y)/2 = 4
2x + y = 8
y = 8 – 2x
From (ii), we get
x + 6y = 15 …(iii)
Substituting y = 8 – 2x in (iii), we get
x + 6 (8 – 2x) = 15
⇒ x + 48 – 12x = 15
⇒ -11x = -33
⇒ x = -33/-11 = 3
Putting x = 3, in y = 8 – 2x, we get
y = 8 – 2×3
= 8 – 6 = 2
⇒ y = 2
Hence, solution of the given system of equation is x= 3 , y = 2.
10. x + 2y = 3/2
2x + y = 3/2
Solution
The given system of equation is
x + 2y = 3/2 …(i)
2x + y = 3/2 …(ii)
Let us eliminate y from the given equations. The Coefficients of y in the given equations are 2 and 1 respectively. The L.C.M of 2 and 1 is 2. So, we make the coefficient of y equal to 2 in the two equations.
Multiplying (i) by 1 and (ii) by 2, we get
x + 2y = 3/2 …(iii)
4x + 2y = 3 …(iv)
Subtracting (iii) from (iv), we get
4x – x + 2y – 2y = 3 – 3/2
⇒ 3x = (6 – 3)/2
⇒ 3x = 3/2
⇒ x =3/2×3
⇒ x = ½
Putting x = ½, in equation (iv) , we get
4×1/2 + 2y = 3
⇒ 2 + 2y = 3
⇒ 2y = 3- 2
⇒ y = ½
Hence, solution of the given system of equation is x = 1/2, y = 1/2.
11. √2x + √3y = 0
√3x - √8 y = 0
Solution
√2x + √3y = 0 ...(i)
√3x - √8y = 0 …(ii)
From equation (i), we obtain:
x = −√3y/√2 …..(iii)
Substituting this value in equation (ii), we obtain:
√3(−√3y/√2) - √8y = 0
-3y/√2 - 2√2y = 0
y(-3/√2 - 2√2) = 0
y = 0
Substituting the value of y in equation (iii), we obtain:
x = 0
∴ x = 0, y = 0
12. 3x – (y+7)/11 + 2 = 10
2y + (x+ 11)/7 = 10
Solution
The given systems of equation is
3x – (y+7)/11 + 2 = 10 …(i)
2y + (x + 11)/7 = 10 …(ii)
From (i), we get
(33x – y – 7 + 22)/11 = 10
⇒ 33x – y + 15 = 10×11
⇒ 33x + 15 – 110 = y
⇒ y = 33x – 95
From (ii) we get
(14y + x + 11)/7 = 109
⇒ 14y + x + 11 = 10×7
⇒ 14y +x + 11 = 70
⇒ 14y + x = 70 – 11
⇒ 14y + x = 59 …(iii)
Substituting y = 33x – 95 in (iii), we get
14(33x – 95) +x = 59
⇒ 462x – 1330 + x = 59
⇒ 463x = 59 + 1330
⇒ 463x = 1389
⇒ x = 1389/463 = 3
Putting x = 3, in y = 33x – 95 , we get
y = 33×3 – 95
⇒ y = 99 – 95 = 4
⇒ y = 4
Hence, solution of the given system of equation is x = 3, y = 4.
13. 2x – 3/y = 9
3x + 7/y = 2 y≠0
Solution
The given system of equation is
2x – 3/y = 9 …(i)
3x + 7/y = 2, y ≠ 0 …(ii)
Taking 1 /y = u, the given equations becomes
2x – 3u = 9 …(iii)
3x + 7u = 2 …(iv)
From (iii), we get
2x = 9 + 3u
⇒ x = (9 + 3u)/2
Substituting x = (9+3u)/2 in (iv) , we get
3(9 +3u)/2 + 7u = 2
⇒ (27+ 9u + 14u)/2 = 2
⇒ 27 + 23u = 2×2
⇒ 23u = 4 – 27
⇒ u = -23/23 = - 1
Hence, y = 1/u = 1/-1 = - 1
Putting u = -1 in x = (9+ 3u)/2, we get
x = (9 + 3×-1)/2 = (9 -3)/2 = 6/2 = 3
⇒ x = 3
Hence, solution of the given system of equation is x = 3, y = -1.
14. 0.5x + 0.7y = 0.74
0.3x + 0.5y = 0.5
Sol : The given systems of equations is
0.5x + 0.7y = 0.74 …(i)
0.3x + 0.5y = 0.5 …(ii)
Multiplying (i) and (ii) by 100, we get
50x + 70y = 74 …(iii)
30x + 50y = 50 …(iv)
From (iii), we get
50x = 74 – 70y
x = (74 – 70y)/50
Substituting x = (74 – 70y)/50 in equation (iv), we get
30(74 – 70y)/50 + 50y = 50
⇒ 3(74 – 70y)/5 + 50y = 50
⇒ (222 – 210y)/5 + 50y = 50
⇒ 222 – 210y + 250y = 250
⇒ 40y = 250 – 222
⇒ 40y = 28
⇒ y = 28/40 = 14/20 = 7/10 = 0.7
Putting y = 0.7 in x = (74 – 70y)/50 , we get
x = (74 – 70×0.7)/50
= (74 – 49 )/50
= 25/50 = 1/2 = 0.5
Hence, solution of the given system of equation is x = 0.5, y = 0.7
15. 1/7x + 1/6y = 3
1/2x – 1/3y = 5
Solution
1/7x + 1/6y = 3 …(i)
1/2x – 1/3y = 5 …(ii)
Multiplying (ii) by 1/2, we get
1/7x + 1/6y = 3
⇒ (4+7)/28x = (6+5)/2
⇒ 11/28x = 11/2
⇒ x = (11×2)/(28×11) = 1/14
When x = 1/14, we get
1/7(1/14) + 1/6y = 3 (Using (i)]
⇒ 2 + 1/6y = 3
⇒ 1/6y = 3 – 2 = 1
⇒ y = 1/6
Thus , the solution of given equation is x = 1/14 and y = 1/6.
16. 1/2x + 1/3y = 2
1/3x + 1/2y = 13/6
Solution
Let 1/x = u and 1/y = v, the given equations become
u/2 + v/3 = 2
⇒ (3u + 2v)/6 = 2
⇒ 3u + 2v = 12 …(i)
And, u/3 + v/2 = 13/6
⇒ (2u + 3v)/6 = 13/6
⇒ v = 6/2 = 3
Hence, x = 1/u = 1/2 and y = 1/v = 1/3
So, the solution of the given system equation is x = 1/2, y = 1/3.
17. (x +y)/xy = 2
(x – y)/xy = 6
Solution
The given system of equation is
(x + y)/xy = 2
⇒ x/xy + y/xy = 2
⇒ 1/y + 1/x = 2 ….(i)
And, (x – y)/xy = 6
⇒ x/xy – y/xy = 6
⇒ 1/y – 1/x = 6 ….(ii)
Taking 1/y = v and 1/x = u, the above equations become
v + u = 2 ….(iii)
v – u = 6 ….(iv)
Adding equation (iii) and equation (iv) , we get
v+ u + v – u = 2 + 6
⇒ 2v = 8
⇒ v = 8/2 = 4
Putting v = 4 in equation (iii), we get
4 + u = 2
⇒ u = 2 – 4 = - 2
Hence, x = 1/u = 1/ - 2 = -1/2 and y = 1/v = 1/4
So, the solution of the given system of equation is x = -1/2, y = 1/4
18. 15/u + 2/v = 17
Solution
Let 1/u = x and 1/v = y, then the given system of equations become
15x + 2y = 17 ….(i)
x + y = 36/5 …(ii)
From (i), we get
2y = 17 – 5x
⇒ y = (17 – 15x)/2
Substituting y = (17 – 15x)/2 in equation (ii), we get
x + (17 – 15x)/2 = 36/5
⇒ (2x + 17 – 15x)/2 = 36/5
⇒ (-13x + 17)/2 = 36/5
⇒ 5(-13x + 17) = 36×2
⇒ -65x + 85 = 72
⇒ -65x = 72 – 85
⇒ -65x = -13
⇒ x = -13/-65 = 1/5
Putting x = 1/5 in equation (ii), we get
1/5 + y = 36/5
⇒ y = 36/5 – 1/5
⇒ y = 35/5 = 7
Hence u = 1/x = 5 and v = 1/y = 1/7 .
So, the solution of the given system of equation is u = 5, v = 1/7.
19. 3/x – 1/y = - 9
2/x + 3/y = 5
Solution
Let 1/x = u and 1/y = v, Then, the given system of equations becomes
3u – v = -9 ...(i)
2u + 3v = 5 …(ii)
Multiplying equation (i) by 3 and equation (ii) by 1, we get
9u – 3v = -27 …(iii)
2u + 3v = 5 …(iv)
Adding equation (i) and equation (ii), we get
9u + 2u – 3v + 3v = -27 + 5
⇒ 11u = -22
⇒ u = -22/11 = -2
Putting u = -2 in equation (iv), we get
2×(-2) + 3v = 5
⇒ -4 + 3v = 5
⇒ 3v = 5 + 4
⇒ v = 9/3 = 3
Hence, x = 1/u = 1/-2 = -1/2 and y = 1/v = 1/3.
So, the solution of the given system of equation is x = -1/2, y = 1/3.
20. 2/x + 5/y = 1
60/x + 40y = 19, x ≠0, y ≠0
Solution
Taking 1/x = u and 1/y = v, the given becomes
2u + 5v = 1 …(i)
60u + 40u = 19 …(ii)
Let us eliminate ‘u’ from equation (i) and (ii), multiplying equation (i) by 60 and equation (ii) by 2, we get
120u +300v = 60 …(iii)
120u + 80v = 38 …(iv)
Subtracting (iv) from (iii), we get
300v – 80v = 60 – 38
⇒ 220v = 22
⇒ v = 22/220 = 1/10
Putting v = 1/10 in equation (i), we get
2u + 5×1/10 = 1
⇒ 2u + 1/2 = 1
⇒ 2u = 1 – 1/2
⇒ 2u = (2-1)/2 = 1/2
⇒ 2u = 1/2
⇒ u = 1/4
Hence, x = 1/u = 4 and y = 1/v = 10
So, the solution of the given system of equation is x = 4 , y = 10.
21. 1/5x + 1/6x = 12
1/3x – 3/7y = 8, x ≠0, y≠0
Solution
Taking 1/x = u and 1/y = v, the given equations become
u/5 + v/6 = 12
⇒ (6u + 5v)/30 = 12
⇒ 6u + 5v = 360 …(i)
And u/3 – 3v/7 = 8
⇒ (7u + 9v)/21 = 8
⇒ 7u – 9v = 168 …(ii)
Let us eliminate ‘v’ from equation (i) and (ii), multiplying equation (i) by 9 and equation (ii) by 5, we get
54u + 45v = 3240 …(iii)
35u – 45v = 840 …(iv)
Adding equation (i) adding equation (ii), we get
54u + 35u = 3240 + 840
⇒ 89u = 4080
⇒ u = 4080/89
Putting u = 4080/89 in equation (i) , we get
6× 4080/89 + 5v = 360
⇒ 24480/89 + 5v = 360
⇒ 5v = 360 – 24480/89
⇒ 5v = (32040- 24480)/89
⇒ 5v = 7560/89
⇒ v = 7560/5×89
⇒ v = 1512/89
Hence, x = 1/u = 89/4080 and y = 1/v = 89/1512
So, the solution of the given system of equation is x = 89/4080, y = 89/1512
22. 2/x + 3/y = 9/xy
4/x + 9/y = 21/xy , where x ≠0, y ≠ 0
Solution
The system of given equation is
2/x + 3/y = 9/xy …(i)
4/x + 9/y = 21/xy , where x ≠0, y = 0 …(ii)
Multiplying equation (i) adding equation (ii) by xy, we get
2y + 3x = 9 …(iii)
4y + 9x = 21 …(iv)
From (iii), we get
3x = 9 – 2y
⇒ x = (9 – 2y)/3
Substituting x = (9 – 2y)/3 in equation (iv) , we get
4x + 9(9-2y)/3 = 21
⇒ 4y + 3(9 -2y) = 21
⇒ 4y + 27 – 6y = 21
⇒ -2y = 21 – 27
⇒ -2y = -6
⇒ y =3
Putting y = 3 in x = (9-2y)/3 , we get
x = (9 – 2×3)/3
= (9-6)/3
= 3/3 = 1
Hence, solution of the system of equation is x = 1, y = 3
23. 6/(x+y) = 7/(x – y) + 3
1/2(x+ y) = 1/3(x – y), where x + y ≠ 0 and x – y ≠ 0
Solution
Let 1/x + y = u and 1/x – y = v. Then the given system of equation becomes
6u = 7v + 3
⇒ 6u – 7v = 3 …(i)
And, u/2 =v/3
⇒ 3u = 2v
⇒ 3u – 2v = 0 …(ii)
Multiplying equation (ii) by 2, and equation (i) by 1, we get
6u – 7v = 3 …(iii)
6u – 4v = 0 …(iv)
Subtracting equation (iv) from equation (iii), we get
- 7 + 4v = 3
⇒ -3v = 3
⇒ v = -1
Putting v = -1 in equation (ii), we get
3u – 2×(-1) = 0
⇒ 3u + 2 = 0
⇒ 3u = -2
⇒ u = -2/3
Now, u = -2/3
⇒ 1/(x +2) = -2/3
⇒ x + y = -3/2 …(v)
And, v = - 1
⇒ 1/(x –y) = -1
⇒ x –y = -1 …(vi)
Adding equation (v) and equation (vi), we get
2x = -3/2 – 1
⇒ 2x = (-3-2)/2
⇒ 2x = -5/2
⇒ x =-5/4
Putting x = -5/4 in equation (vi) , we get
-5/4 – y = -1
⇒ -5/4 + 1 = y
⇒ (-5+4)/4 = y
⇒ -1/4 = y
⇒ y = -1/4
Hence, solution of the system of equation is x = -5/4, y = -1/4.
24. xy/(x+y) = 6/5
xy/(y-x) = 6
Solution
25. 22/(x+y) + 15/(x -y) = 5
55/(x +y) + 45/(x -y) = 14
Solution

26. 5/(x+y) - 2/(x-y) = -1
15/(x+ y) + 7/(x -y) = 10
Solution

Hence, solution of the given system of equation is x = 3, y = 2 .
27. 3/(x+y) + 2/(x-y) = 2
9/(x+y) - 4/(x-y) = 1
Solution

28. 1/2(x+2y) + 5/3(3x-2y) = -3/2
5/4(x+2y) - 3/5(3x-2y) = 61/60
Solution

29. 5/(x+1) - 2/(y-1) = 1/2
10/(x+1) + 2/(y-1) = 5/2, where x ≠ -1 and y ≠ 1
Solution

30. x+y = 5xy
3x + 2y = 13xy
Solution

31. x+ y = 2xy
(x-y)/xy = 6 x ≠ 0, y ≠ 0
Solution

32. 2(3u - v) = 5uv
2(u + 3v) = 5uv
Solution

33. 2/(3x + 2y) + 3/(3x - 2y) = 17/5
5/(3x + 2y) + 1/(3x -2y) = 2
Solution

34. 4/x + 3y = 14
3/x -4y = 23
Solution

35. 99x + 101y = 499
101x + 99y = 501
Solution

36. 23x - 29y = 98
29x - 23y = 110
Solution
The given system of equation is
23x - 29y = 98 ...(i)
29x - 23y = 110 ...(ii)
37. x - y +z = 4
x - 2y -2z = 9
2x + y + 3z = 1
Solution
We have,
x - y +z = 4 ...(i)
x - 2y -2z = 9 ...(ii)
2x + y + 3z = 1 ...(iii)
From equation (i) , we get
z = 4 - x + y
⇒ z = -x + y + 4
Subtracting the value of z in equation (ii), we get
38. x - y +z = 4
x + y + z = 2
2x + y - 3z = 0
Solution
We have,
x - y +z = 4 ...(i)
x + y + z = 2 ...(ii)
2x + y - 3z = 0 ...(iii)
From equation (i), we get
39. 44/(x+y) + 30/(x-y) = 4
55/(x+y) + 40/(x-y) = 13
Solution
Let 1/(x+ y) = u and 1/(x -y) = v.
Then, the system of the given equations becomes
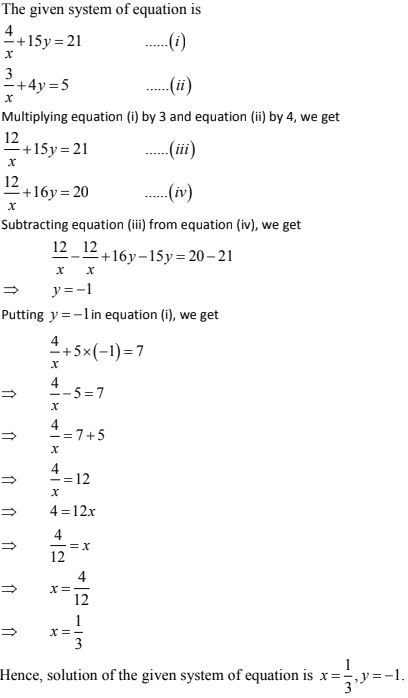
40. 4/x + 15y = 21
3/x + 4y = 5
Solution
41. 2(1/x) + 3(1/y) = 13
5(1/x) - 4(1/y) = -2
Solution

42. 5/(x-1) + 1/(y-2) = 2
6/(x-1) - 3/(y-2) = 1
Solution
5/(x-1) + 1/(y-2) = 2 ...(i)
6/(x-1) - 3/(y-2) = 1 ...(ii)
Let 1/(x - 1) = u, 1/(y-2) = v
So, our equations become
5u+ v = 2 ...(iii)
6u - 3v = 1 ...(iv)
From equation (iii),
5u + v = 2
v = 2 - 5u ...(v)
Putting value of v in (iv)
6u - 3v = 1
6u - 3(2 - 5u) = 1
6u - 6 + 15u = 1
21u = 1+6
u = 7/21 = 1/3
Putting u = 1/3 in equation (v)
v = 2 - 5u
= 2 - 5×1/3
= 2 - 5/3
= (6-5)/3 = 1/3
Now, 1/(x-1) = u
1/(x -1) = 1/3
⇒ x -1 = 3
⇒ x = 4
and , 1/(y - 2) = v
⇒ 1/(y-2) = 1/3
⇒ y - 2 = 3
⇒ y = 5
Hence, solution of the given system of equation is x = 4, y = 5.
43. 10/(x+y) + 2/(x-y) = 4
15/(x+y) - 5/(x-y) = -2
Solution

44. 1/(3x+y)+ 1/(3x-y) = 3/4
1/2(3x+y) - 1/2(3x- y) = -1/8
Solution

45. 2/√x + 3/√y = 2
4/√x - 9/√y = -1
Solution

46. (7x - 2y)/xy = 5
(8x + 7y)/xy = 15
Solution

47. 152x - 378y = -74
-378x + 152y = -604
Solution
152x - 378y = -74 ...(i)
-378x + 152y = -604 ...(ii)
Adding the equations (i) and (ii), we obtain:
-226x - 226y = -678
⇒ x + y = 3 ...(iii)
Subtracting the equation(ii) from equation (i) , we obtain
530x - 530y = 530
⇒ x - y = 1 ...(iv)
Adding equations (iii) and (iv), we obtain:
2x = 4
x = 2
Substituting the value of x in equation (iii) , we obtain:
y = 1






