RD Sharma Solutions for Class 10 Chapter 2 Polynomials Exercise 2.3

RD Sharma Solutions Chapter 2 Polynomials Exercise 2.3 Class 10 Maths

Chapter Name	RD Sharma Chapter 2 Polynomials
Book Name	RD Sharma Mathematics for Class 10
Other Exercises	Exercise 2.1 Exercise 2.2
Related Study	NCERT Solutions for Class 10 Maths

Exercise 2.3 Solutions

1. Apply division algorithm to find the quotient q(x) and remainder r(x) on dividing f(x) by g(x) in each of the following:

(i) f(x) = x³ - 6x² + 11x - 6,  g(x) = x² + x + 1
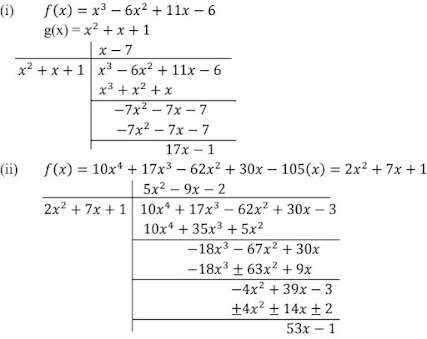
(ii)  f(x) = 10x⁴ + 17x³ - 62x²  + 30x - 105,  g(x) = 2x² + 7x + 1 
(iii) f(x) = 4x³ + 8x² + 8x + 7, g(x) = 2x² - x + 1 
(iv) f(x) = 15x³ - 20x² + 13x - 12;  g(x) = x² - 2x + 2

Solution 

3. Obtain all zeros of the polynomial f(x) = 2x⁴ + x³ - 14x² - 19x - 6, if two of its zeros are -2 and -1. 

Solution

f(x) = 2x⁴ + x³ - 14x² - 19x - 6 
If the two zeroes of the polynomial are -2 and -1, then its factors are (x+2) and (x+1).
(x+2)(x+1) = x² + x + 2x = x² + 3x + 2 

∴ 2x⁴ + x³ - 14x² - 19x - 6 
= (2x² - 5x - 3)[x² + 3x + 2] 
= [2x + 1][x - 3][x+2][x+1]
∴ zero all x = -1/2, 3, -2, -1 

4. Obtain all zeros of f(x) = x³ + 13x² + 32x + 20, if one of its zeros is -2. 

Solution

(x² + 11x + 10) = x² + 10x + x + 20(x + 10) = (x+ 1)(x+10)
∴ The zeroes of the polynomial are -1, -10, -2. 

5. Obtain all zeros of the polynomial f(x) = x⁴ - 3x² = x² + 9x - 6 if two of its zeros are -√3, and √3. 

Solution 

(x² - 3)(x² - 3x + 2) = (x + √3)(x - √3)(x² - 2x - x + 2)
= (x + √3)(x - √3)(x-2)(x-2)
Zeroes are - √3, √3, 1, 2 

6. Find all zeros of the polynomial f(x) = 2x⁴ - 2x³ - 7x² + 3x + 6, if its two zeroes are -√(3/2) and √(3/2).

Solution

If the zeroes of the polynomial are -√(3/2) and √(3/2)

7. What must be added to the polynomial f(x) = x⁴ + 2x³ - 2x² + x - 1 so that the resulting polynomial is exactly divisible by x² + 2x -3 ? 

Solution

we must add x - 2 in order to get the resulting polynomial exactly divisible by x² + 2x - 3 

8. What must be subtracted from the polynomial x⁴ + 2x³ - 13x² - 12x + 21, so that the resulting polynomial is exactly divisible by x² - 4x + 3 ?

Solution

We must subtract [2x - 2] + 10m the given polynomial so as to get the resulting polynomial exactly divisible by x² - x + 3. 

9. Find all the zeroes of the polynomial x⁴ + x³ - 34x² - 4x + 120, if two of its zeroes are 2 and -2.

Solution

f(x) = x⁴ + x³ - 34x² - 4x + 120

⇒ x = -2 is a solution 
x = -2 is a factor 
x = -2 is a solution 

x = +2 is a factor 

here,
(x - 2)(x + 2) is a factor of f(x) 

x² - 4 is a factor 

Hence, x⁴ + x³ - 34x² - 4x + 120 = (x² -4)(x² + x -30)
x⁴ + x³ - 34x² - 4x + 120 = (x² - 4)(x² + 6x - 5x - 30)
⇒ x⁴ + x³ - 34x² - 4x + 120 = (x² -4)[x(x+6) - 5(x + 6)]
⇒ x⁴ + x³ - 34x² - 4x + 120 = (x² -4)(x + 6) (x- 5)
Other zeroes are 

x + 6 = 0 
⇒ x = -6 

x - 5 = 0 
⇒ x = 5 
Se of zeroes for f(x) [2, -2, 6, 5]

10. Find all zeros of the polynomial 2x⁴ + 7x³ - 19x² - 14x + 30,  if two of its zeros are √2 and - √2. 

Solution

f(x) = 2x⁴ + 7x³ - 19x² - 14x + 30

x = √2 is a solution

x - √2 is a solution

x + √2 is a factor

Here , (x + √2)(x -√2) is a factor of f(x) 
x² - 2 is a factor of f(x) 

Hence, 2x⁴ + 7x³ - 19x² - 14x + 30 = (x² - 2)(2x² + 7x - 15)
= (x² - 2)(2x² + 10x - 3x - 15)
= (x² - 2)[2x(x+ 5) - 3(x + 5)]
= (x² - 2)(x + 5)(x - 3)
Other zeroes are : 
x + 5 = 0 
⇒ x = -5 
2x - 3 = 0 
⇒ 2x = 3 
⇒ x = 3/2
Hence, the set of zeroes for f(x) {-5, 3/2, √2, -√2}

11. Find all the zeroes of the polynomial 2x³ + x² - 6x - 3,  if two of its zeros are -√3 and √3. 

Solution

f(x) =   2x³ + x² - 6x - 3
x = -√3 is a solution 

x + √3 is a factor 
x = √3 is a solution 

x - √3  is a factor 

Here, (x +√3)(x-√3) is a factor of f(x) 
x² - 3 is a factor of f(x) 

Hence, 2x³ + x² - 6x - 3 = (x² - 3)(2x - 1)
Other zeroes of f(x) is 2x + 1  = 0 
x = -1/2 
Set of zeroes {√3, -√3, -1/2}

12. Find all the zeros of the polynomial x³ + 3x² -2x - 6, if two of its zeros are -√2 and √2. 

Solution

Since, -√2 and √2 are zeroes of polynomial f(x) = x³ + 3x² -2x - 6 
(x + √2)(x - √2) = x² - 2 is a factor of f(x) 
Now we divide f(x) = x³ + 3x² - 2x - 6 by 
g(x) = x² - 2 to b find the other zeroes of f(x) 

By division algorithm, we have 
⇒ x³ + 3x² - 2x - 6 = (x² - 2)(x+ 3)
⇒ x³ + 3x² - 2x - 6 = (x + √2)(x - √2(x + 3)
Here the zeroes of the given polynomials are -√2, √2 and -3