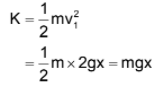Revision Notes for Work, Energy and Power Class 10 Physics ICSE

ICSE Revision Notes for Work, Energy and Power Class 10 Physics

Chapter Name	Work, Energy and Power
Topics Covered	Work Power Energy Work-Energy Theorem Law of Conservation of Energy
Related Study	Selina Physics ICSE Solutions for Class 10 Frank ICSE Solutions for Class 10 Physics

Work 

• Work is any physical or mental activity which one does to perform daily tasks. However, in scientific parlance, work is done when a force produces motion in an object. 
• The amount of work depends on two factors:
  ▪ The magnitude and direction of force applied to an object
  ▪ The distance/displacement through which the object moves 
• The amount of work done by a force in moving a body is equal to the product of the force and the displacement of the point of application of the force in the direction of force.
  Work = Force×Displacement
  W = F × s 
• Work is a scalar quantity.
  
• Thus, the expression of work is
  W = Fs cosθ 
• Thus, the amount of work done is the product of force, displacement and the cosine of the angle between the force and displacement. 
• If the displacement is in the direction of the force, i.e. θ = 0°, then the work done is
  W =  F × s . This work is maximum and positive. 
• If the displacement is normal to the direction of the force, i.e. θ = 90°, then the work done is
  W = 0. Thus, no work is done. 
• If the displacement is zero, then the work done is zero. This is the case when a body is performing circular motion. 
• If the displacement is in the direction opposite to that of the force, i.e. θ = 180°, then the work done is
  W = - F × s.
  This work is minimum and negative.

Work Done by Gravity 

• If a body of mass m moves down from a height h, then the force of gravity or weight acts on the body through a displacement h. 
• Thus, the work done by the force of gravity is
  W = mg × h 
• Similarly, if the body is thrown up to a height h, then the work done by gravity is
  W = - mg × h 
• The SI unit of work is newton metre (N m) or joule (J). 
• One joule of work is said to be done when a force of one newton displaces the body through a distance of one metre in its direction. 
• The CGS unit of work is erg. 
• One erg of work is said to be done when a force of one dyne displaces the body through a distance of one centimetre in its direction.
• 1 joule = 10⁷ erg 

Power: Rate of Doing Work 

• Power is defined as the rate of doing work or the rate of transfer of energy.
  P = W/t
• Power is a scalar quantity. 
• If displacement is at an angle θ, then the power is
  W Fs cosθ
  P = W/t = Fs cosθ/t
  = Fv cos θ
• Its SI unit is watt (W) or joule per second (J/s), and its CGS unit is erg per second (erg/s). 
• Another unit of power is kilowatt (kW).
  ▪ 1 kW = 1000 W
  ▪ 1 MW = 10⁶ W 
• 1 horsepower = 746 W = 0.746 kW 

Energy 

• We can define energy as the capacity to do work. 
• The amount of energy possessed by a body is the amount of work it can do when that energy is released. 
• Energy is a scalar quantity. 
• The SI unit of energy is the same as the unit of work, i.e. joule (J), and its CGS unit is erg. 
• Another unit of energy is watt hour or kilowatt hour. 
• kW h = 3.6 × 10⁶ J = 3.6 MJ
• The commercial unit of electric energy is kilowatt hour (kW h), commonly known as unit.
• 1 kW h = 3.6×10 J = 3.6 MJ
  ▪ Heat energy is usually measured in calorie. One calorie is the energy required in raising the temperature of 1 g of water through 1°C.
  ▪ 1 J = 0·24 calorie
  ▪ 1 calorie = 4·18 J
  ▪ 1 kilocalorie = 1000 calorie = 4180 J
  ▪ 1 eV is the energy gained by an electron when it is accelerated through a potential difference of 1 volt
  ▪ 1 eV = 1·6 × 10⁻¹⁹ J 

Mechanical Energy 

• The energy possessed by a body due to its state of rest or of motion is called mechanical energy. 
• The total mechanical energy of a body is equal to the sum of its kinetic energy and potential energy.

Kinetic Energy 

• The energy possessed by a body by virtue of its state of motion is called kinetic energy. It is denoted by ‘K’. 
• Suppose a body of mass m is moving with a velocity ‘v’. It is brought to rest by applying a constant opposing force F. Let ‘a’ be the uniform retardation produced by the force, and the body travels a distance ‘S’ before coming to rest.
  Kinetic energy = Work done by retarding force in stopping it
  = Retarding force × displacement
  = F × S
  = ma × S
• Thus, the kinetic energy is given as
  K = 1/2 mv²
• The kinetic energy and momentum are related as
  

Work–Energy Theorem 

• According to the work–energy theorem, the work done by a force on a moving body is equal to the increase in its kinetic energy.
  W = ½ mv² - ½ mu² = k_f - k_i
• W = Final kinetic energy – Initial kinetic energy 
• Thus, the work done is the increase in kinetic energy. 

Forms of Kinetic Energy 

• The motion of a body in a straight line path is called translational motion, and the kinetic energy of the body due to motion in a straight line is called translational kinetic energy.
• When a body rotates about an axis, the motion is called rotational motion, and the kinetic energy of the body due to rotational motion is called rotational kinetic energy or simply rotational energy.
• When a body moves to and fro about its mean position, the motion is called vibrational motion. The kinetic energy of the body due to its vibrational motion is called vibrational kinetic energy or simply vibrational energy.

Potential Energy 

• The energy possessed by a body by virtue of its specific position or changed configuration is called potential energy. It is denoted by ‘U’. 
• The potential energy possessed by a body due to its position relative to the centre of the Earth is called its gravitational potential energy.
• Larger the distance of the body from the centre of the Earth, greater is its gravitational potential energy. 
• The potential energy possessed by a body in the deformed state due to change in its configuration is called elastic potential energy. It is equal to the amount of work done in deforming the body or in changing the configuration of the body. 

Gravitational Potential Energy 

• The gravitational potential energy of a body at a height above the ground is measured by the amount of work done in lifting it up to that height against the force of gravity.
• Let a body of mass m be lifted from the ground (or Earth surface) to a vertical height h. The work W done on the body in lifting it to a height h is
  W = Force of gravity (mg) x displacement (h) =mgh 
• This work is stored in the body when it is at a height h in the form of its gravitational potential energy. Thus, gravitational energy is U = mgh. 
• Thus, when a body is thrown vertically upwards, its potential energy increases. Similarly, when a body is dropped from a height, its potential energy decreases.
• Potential energy changes into kinetic energy whenever it is put to use.
• When the string of a bow is pulled, some work is done which is stored in the deformed state of the bow in the form of its elastic potential energy. On releasing the string, the potential energy of the bow changes into the kinetic energy of the arrow which helps to move it forward.

Different forms of Energy 

• The energy radiated by the Sun is called solar energy. 
• Several devices are available to use solar energy. These devices are solar panels, solar furnaces, solar cells etc. 
• The energy released on burning coal, oil, wood or gas is heat energy. 
• Light energy is the form of energy which helps other objects to be seen. 
• The Sun is the natural source of light energy. The moon reflects light from the Sun. Other sources such as fire, candle, tube light, bulb etc. provide light energy. 
• The energy possessed by fossil fuels such as coal, petroleum and natural gas is called chemical energy or fuel energy. 
• The energy possessed by running water is called hydro energy. It is used to generate electricity in hydroelectric power stations. 
• When two dry bodies are rubbed together, they get charged due to the movement of free electrons from one body to the other. Thus, they possess electrical energy. An electric cell is a source of electrical energy. 
• The energy released during the process of nuclear fission and fusion is called nuclear or atomic energy. 
• The energy released in nuclear disintegrations in the interior of the Earth gets stored deep inside the Earth and is called geothermal energy. This energy heats up the underground water to produce natural steam. 
• The energy possessed by fast-moving air is called wind energy. This energy is used in driving a wind mill.
• A vibrating body possesses sound energy. It is sensed by our ears. When the disturbance produced in atmospheric air layers by a vibrating body reaches our ears and produces vibrations in the ear membrane, sound is heard. 
• The energy possessed by a magnet due to which it can attract iron filings is called magnetic energy. An electromagnet has magnetic energy. 
• The energy possessed by a body due to its state of rest or of motion is called mechanical energy. A body at a height, a moving body, a stretched bow etc. have mechanical energy. 

Conversion of One form of Energy into Another 

• In a hydroelectric power station water stored in a dam has potential energy which is converted to kinetic energy while falling and then this mechanical energy is converted to electrical energy by the generator. 
• In an electric motor, the electrical energy is passed through the coil which is freely suspended between the poles of a magnet. This causes the coil to rotate converting the electrical to mechanical energy. 
• In a loudspeaker electrical energy is converted to sound energy. 
• When a candle burns the chemical energy inside the wax gets converted to light energy. 
• In a photocell, light energy incident on gets converted to electrical energy. 
• In automobiles, the chemical energy of petrol is used to run the engine and convert to mechanical energy of the vehicle. 
• Many more such conversions are possible, viz. Heat to electrical, sound to electrical, electrical to chemical, light to chemical, heat to mechanical, etc. 

Law of Conservation of Energy

• According to the law of conservation of energy, energy can neither be created nor be destroyed. It only changes from one form to another.
• The total mechanical energy of an isolated system at any instant is equal to the sum of its kinetic energy and potential energy.
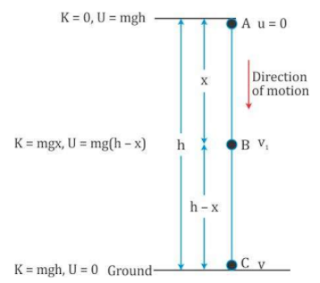
  K + U = Constant: Theoretical Verification 
• Consider a body of mass m freely falling under gravity from a height h.
  

At position A: Total energy = K + U = mgh 

At position B: 

Kinetic energy is 

Potential energy is = mg (h - x) 

Hence, total energy = K + U = mgx + mg(h - x) = mgh 

At position C: 

Kinetic energy is 

Potential energy is 0. 

Hence, total energy = K + U = mgh + 0 = mgh 

• Thus, the mechanical energy always remains constant. Hence, mechanical energy is conserved.

Application of Law of Conservation of Energy to a Simple Pendulum

• At the resting position, the bob of the pendulum has zero potential energy. When the bob is displaced from its resting position, it gets raised by a vertical height h, so its potential energy increases by mgh if m is the mass of the bob. 
• On releasing the bob from a height, it moves back to its initial position. Its vertical height decreases from h to zero, so its potential energy decreases from mgh to zero, and it gets converted into kinetic energy,
  i.e. ½ mv²= mgh.
• Velocity of the bob =
  
• At an intermediate position, the bob has both kinetic energy and potential energy, but the sum of both remains constant throughout the swing.