ICSE Revision Notes for Motion in One Dimension Class 9 Physics

Chapter Name

Motion in One Dimension

Topics Covered

Rest and Motion
Distance and Displacement
Speed and Velocity
Acceleration
Graphical Representation of Linear Motion
Motion under Gravity
Equations of Motion

Related Study

Rest and Motion

A body is said to be at rest if its position with respect to its surrounding does not change.
A body is said to be in motion if its position changes with respect to its surroundings.
One dimensional motion: When a body moves along a straight line path, its motion is said to be one dimensional motion. It is also called motion in a straight line or rectilinear motion.
Representation of one dimensional motion: The path of straight line motion is represented by a straight line parallel to the x-axis.

Distance and Displacement

The total length of the path travelled by a body is called the distance travelled by that body. This path may or may not be straight.
It is a scalar quantity and is represented by S. Its SI unit is metre (m).
The shortest distance from the initial to the final position of the body is called magnitude of displacement. Its direction is from the initial to the final position.
It is a vector quantity and is represented by _S^r. Its SI unit is also metre (m).

Distinction between Distance and Displacement

Distance	Distance
It is the length of path travelled by an object in a certain time.	It is the shortest distance between the initial and final positions.
It is a scalar.	It is a vector.
It depends on the path followed by the object.	It is independent of the path of the object.
It can be more than or equal to the magnitude of displacement.	Its magnitude can be less than or equal to the distance.

Speed

Speed is defined as ‘the distance travelled by an object per unit time’ or ‘the rate of change of distance with time’.
Speed (v) = Distance (S)/time(t)
The SI unit of speed is metre per second and is written as m s⁻¹.
It is a scalar quantity.

Uniform speed: Uniform speed is possessed by an object when it travels equal distances in equal intervals of time, no matter how small these time intervals may be.

Non-uniform or variable speed: Non-uniform speed is possessed by an object when it travels unequal distances in equal intervals of time, no matter how small these time intervals may be.

Instantaneous speed

When the speed of a body changes continuously with time, its speed at a particular instant is known as instantaneous speed.
The speedometer of a vehicle measures the instantaneous speed.

Average speed

The average speed of a body is the total distance travelled by the body divided by the total time taken to cover this distance.
Average speed = (Total distance travelled)/(Total time taken)

Velocity

The velocity of a body is defined as ‘the distance travelled by an object per unit time in a given direction’.
Velocity = (Distance travelled in a given direction)/(Time taken)
Velocity = Displacement/time
The SI unit of velocity is metre per second and is written as m s⁻¹.
Velocity is a vector quantity.

Uniform velocity

Uniform velocity is possessed by an object when it travels in a specified direction in a straight line and covers equal distances in equal intervals of time, no matter how small these time intervals may be.

Non-uniform or variable velocity

Non-uniform velocity is possessed by an object when it travels in a specified direction in a straight line and covers unequal distances in equal intervals of time.
It is also possessed when the object travels equal distances in equal intervals of time, but the direction does not remain the same.

Instantaneous velocity

When the velocity of a body changes continuously with time, its velocity at a particular instant is known as instantaneous velocity.

Average velocity

The average velocity of a body is the displacement of the body divided by the total time taken to cover the entire journey.
Average velocity = (Displacement)/(Total time taken)

Difference between Speed and Velocity

Speed	Velocity
The distance travelled by a moving object per unit time is speed	The distance travelled by a moving object per unit time in a particular direction is velocity.
It is a scalar.	It is a vector.
It is always positive.	It may be positive or negative depending on the direction of motion.
If the body is moving in a circle, then after one round, the average speed is not zero.	If the body is moving in a circle, then after one round, the average velocity is zero.

Acceleration

∙ Acceleration of a body is defined as ‘the rate of change of its velocity with time’.
Acceleration = (Final velocity - Initial velocity)/(Time taken for change)
a = (v - u)/t
The SI unit of acceleration is metre per second square and is written as m s⁻².
Acceleration is a vector quantity.

Uniform Acceleration

A body is said to possess uniform acceleration if it travels in a straight line and its velocity increases by equal amounts in equal intervals of time.

Non-uniform or variable acceleration

A body is said to possess non-uniform acceleration if its velocity increases by unequal amounts in equal intervals of time.

Acceleration due to gravity

When a body falls freely under the influence of gravity, the acceleration produced in the body is acceleration due to gravity.
It is denoted by the letter ‘g’.

Retardation

When the velocity of a body increases with time, it is called acceleration. However, if the velocity decreases, then it is called retardation, deceleration or negative acceleration.

Graphical Representation of Linear Motion

Displacement–time graph

This graph has displacement along the y-axis and time along the x-axis.
The slope of the displacement–time graph gives the velocity of the body.
If the slope is positive, then the body is moving away from the point where it started. However, if the slope is negative, then the body is moving back towards the starting point.

Case I: For a stationary body.

Case II: For a body moving with uniform velocity.

Case III: For a body moving with variable velocity.

Velocity–time graph

This graph has velocity along the y-axis and time along the x-axis.
The slope of the velocity–time graph gives the acceleration of the body. Also, displacement of the body can be found from this graph.

(a) Finding displacement

Displacement is the product of velocity and time. Hence, the area enclosed by the velocity–time graph will give the displacement of the body.

(b) Finding acceleration

Acceleration is the ratio of velocity to time. Thus, the slope of the graph will give the acceleration of the body.

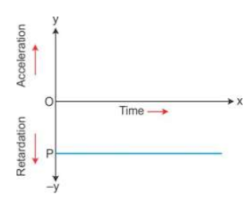
Acceleration–time graph

This graph has acceleration along the y-axis and time along the x-axis.
The change in speed of the body can be found from this graph by finding the area enclosed under the graph.
The following cases are possible:

Case I: For a stationary body or a body moving with uniform acceleration.

The acceleration–time graph is a straight line coinciding with the time axis.

Case II: For a body moving with uniformly increasing velocity.

The acceleration–time graph will be a straight line parallel to the time axis.

Case III: For a body moving with uniformly decreasing velocity.

The acceleration–time graph will be a straight line parallel to the time axis and on the negative acceleration axis.

Motion under Gravity

When a body falls freely on the Earth under the influence of gravity, it is said to be moving with constant acceleration. Its value is 9.8 m/s².
Similarly, when a body is moving upwards against gravity, it is said to be moving with constant retardation. Its value is also 9.8 m/s².

Equations of Motion

The equations of motion are considered for a body moving with uniform acceleration.
The three equations of motion can be derived using the velocity–time graph:

First equation: v = u + at
Second equation: s = ut + 1/2 at²
Third equation: v² = u² + 2as