NCERT Solutions for Chapter 10 Circles Class 9 Maths
Chapter Name | NCERT Solutions for Chapter 10 Circles |
Class | Class 9 |
Topics Covered |
|
Related Study Materials |
|
Short Revision for Ch 10 Circles Class 9 Maths
- A circle is the collection of those points in a plane, whose distances from a fixed point are equal.
- The centre of a circle is a fixed point.
- Radius of a circle is the line segment joining the centre and any point on the circle.
- A circle divides its plane into three parts:
(i) interior of the circle
(ii) The circle and
(iii) Exterior of the circle. - The circular region is the combination of the circle and interior of the circle.
- Diameter of a circle is double of its radius.
- All radii of a circle have the same length.
- All diameters of a circle have the same length.
- A chord of a circle is the line segment joining any two distinct points lying on the circle.
- Diameter of a circle is the longest chord.
- Circumference of a circle is its whole length.
- A segment of a circle is the region between a chord and either of its arcs.
- The arc is a piece of the circle.
- A diameter of a circle divides it into two equal arcs.
- If a circle is divided into two unequal arcs, then the longer one and the shorter one are called major arc and minor arc respectively.
- A sector of a circle is the region between an arc and two corresponding radii.
- Equal chords of circles subtend equal angles at the centre.
- Any chord of a circle is bisected by the perpendicular from the centre.
- The line drawn through the centre of a circle to bisect any chord is perpendicular to that chord.
- There is one and only one circle passing through three given non-collinear points.
- Circumcircle of a triangle is a unique circle passing through all the three vertices of the triangle.
- The radius of a circumcircle is called the circumradius of the triangle.
- The centre of a circumcircle is called the circumcentre of the triangle.
- The distance of a line from a point is the length of the perpendicular drawn from the point to the line.
- Equal chords of a circle (or of congruent circles) are equidistant from the centre (or centres).
- The chords equidistant from the centre of a circle (or from the centres of congruent circles) are equal in length.
- The corresponding arcs of equal chords of a circle are congruent.
- The chords of a circle corresponding to congruent arcs are equal in length.
- Equal arcs (or congruent arcs) of a circle subtend equal angles at the centre.
- The angle subtended by an arc at the centre is double th angle subtended by it at any point on the remaining pa of the circle.
- Angles in the same segment of a circle are equal.
- The points lying on same circle are called concyclic.
- A quadrilateral is said to be cyclic quadrilateral, if all the four vertices lie on the same circle.
- The sum of either pair of opposite angles of a cyclic quadrilateral is 180°.
- An exterior angle of a cyclic quadrilateral is equal to its opposite interior angle.
- Angle in a semicircle is a right angle.
Exercise: 10.1
1. Fill in the blanks:
(i) The centre of a circle lies in ____________ of the circle. (exterior/ interior)
(ii) A point, whose distance from the centre of a circle is greater than its radius lies in __________ of the circle. (exterior/ interior)
(iii) The longest chord of a circle is a _____________ of the circle.
(iv) An arc is a ___________ when its ends are the ends of a diameter.
(v) Segment of a circle is the region between an arc and _____________ of the circle.
(vi) A circle divides the plane, on which it lies, in _____________ parts.
(ii) exterior
(iii) diameter
(iv) semicircle
(v) the chord
(vi) three.
2. Write True or False: Give reasons for your Solutions.
(i) Line segment joining the centre to any point on the circle is a radius of the circle.
(ii) A circle has only finite number of equal chords.
(iii) If a circle is divided into three equal arcs, each is a major arc.
(iv) A chord of a circle, which is twice as long as its radius, is a diameter of the circle.
(v) Sector is the region between the chord and its corresponding arc.
(vi) A circle is a plane figure.
Exercise: 10.2
1. Recall that two circles are congruent if they have the same radii. Prove that equal chords of congruent circles subtend equal angles at their centres.
2. Prove that if chords of congruent circles subtend equal angles at their centres, then the chords are equal.
Exercise: 10.3
1. Draw different pairs of circles. How many points does each pair have in common? What is the maximum number of common points?
2. Suppose you are given a circle. Give a construction to find its centre.
3. If two circles intersect at two points, prove that their centres lie on the perpendicular bisector of the common chord.
Exercise: 10.4
1. Two circles of radii 5 cm and 3 cm intersect at two points and the distance between their centres is 4 cm. Find the length of the common chord.
2. If two equal chords of a circle intersect within the circle, prove that the segments of one chord are equal to corresponding segments of the other chord.
Solution
Construction : Draw OL and OM perpendiculars to chords AB and CD respectively. Join OP.
To prove : AP = DP and PB = CP.
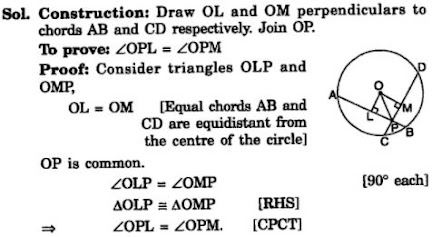
3. If two equal chords of a circle intersect within the circle, prove that the line joining the point of intersection to the centre makes equal angles with the chords.
4. If a line intersects two concentric circles (circles with the same centre) with centre O at A, B, C and D, prove that AB = CD (see Fig. 10.25).
5. Three girls Reshma, Salma and Mandip are playing a game by standing on a circle of radius 5m drawn in a park. Reshma throws a ball to Salma, Salma to Mandip, Mandip to Reshma. If the distance between Reshma and Salma and between Salma and Mandip is 6m each, what is the distance between Reshma and Mandip?
6. A circular park of radius 20m is situated in a colony. Three boys Ankur, Syed and David are sitting at equal distance on its boundary each having a toy telephone in his hands to talk each other. Find the length of the string of each phone.
Exercise: 10.5
1. In Fig. 10.36, A,B and C are three points on a circle with centre O such that BOC = 30° and AOB = 60°. If D is a point on the circle other than the arc ABC, find ADC.
3. In Fig. 10.37, PQR = 100°, where P, Q and R are points on a circle with centre O. Find OPR.
4. In Fig. 10.38, ABC = 69°, ACB = 31°, find BDC.
5. In Fig. 10.39, A, B, C and D are four points on a circle. AC and BD intersect at a point E such that ∠ BEC = 130° and ∠ ECD = 20°. Find BAC.
6. ABCD is a cyclic quadrilateral whose diagonals intersect at a point E. If ∠ DBC = 70°, ∠ BAC is 30°, find ∠ BCD. Further, if AB = BC, find ∠ ECD.
7. If diagonals of a cyclic quadrilateral are diameters of the circle through the vertices of the quadrilateral, prove that it is a rectangle.
8. If the non-parallel sides of a trapezium are equal, prove that it is cyclic.
9. Two circles intersect at two points B and C. Through B, two line segments ABD and PBQ are drawn to intersect the circles at A, D and P, Q respectively (see Fig. 10.40). Prove that ∠ ACP = ∠ QCD.
10. If circles are drawn taking two sides of a triangle as diameters, prove that the point of intersection of these circles lie on the third side.
11. ABC and ADC are two right triangles with common hypotenuse AC. Prove that ∠ CAD = ∠CBD.
12. Prove that a cyclic parallelogram is a rectangle.
Exercise: 10.6
1. Prove that the line of centres of two intersecting circles subtends equal angles at the two points of intersection.
2. Two chords AB and CD of lengths 5 cm and 11 cm respectively of a circle are parallel to each other and are on opposite sides of its centre. If the distance between AB and CD is 6 , find the radius of the circle.
3. The lengths of two parallel chords of a circle are 6 cm and 8 cm. If the smaller chord is at a distance 4 cm from the centre, what is the distance of the other chord from the centre?
4. Let the vertex of an angle ABC be located outside a circle and let the sides of the angle intersect equal chords AD and CE with the circle. Prove that ∠ABC is equal to half the difference of the angles subtended by the chords AC and DE at the centre.
5. Prove that the circle drawn with any side of a rhombus as diameter, passes through the point of intersection of its diagonals.
6. ABCD is a parallelogram. The circle through A, B and C intersect CD (produced if necessary) at E. Prove that AE, = AD.
Solution
ABCD is a parallelogram.
∴ ∠ABC = ∠CDA ...(i) [Opposite angles of a parallelogram]
7. AC and BD are chords of a circle which bisect each other. Prove that (i) AC and BD are diameters; (ii) ABCD is a rectangle.
8. Bisectors of angles A, B and C of a triangle ABC intersect its circumcircle at D, E and F respectively. Prove that the angles of the triangle DEF are 90°–(½)A, 90°–(½)B and 90°–(½)C.
9. Two congruent circles intersect each other at points A and B. Through A any line segment PAQ is drawn so that P, Q lie on the two circles. Prove that BP = BQ.
10. In any triangle ABC, if the angle bisector of ∠A and perpendicular bisector of BC intersect, prove that they intersect on the circumcircle of the triangle ABC.