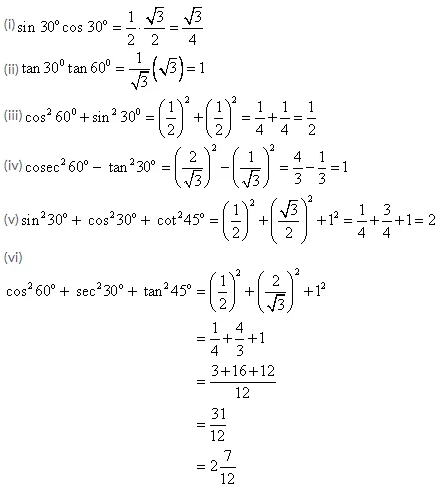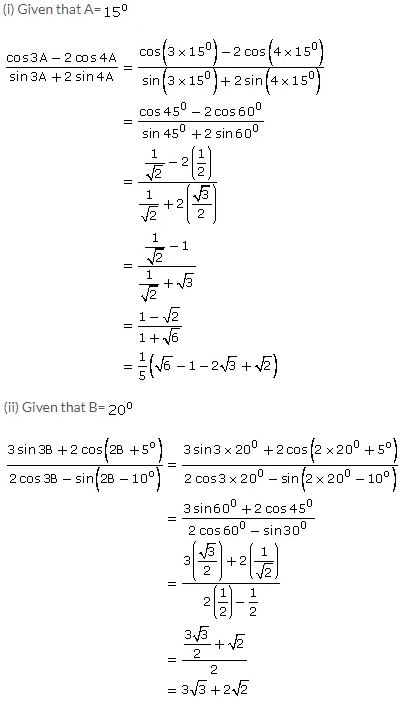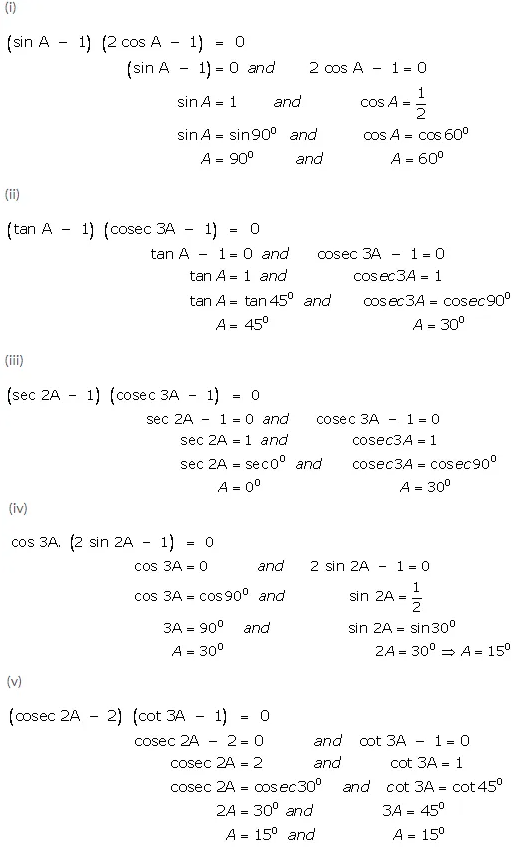Selina Chapter 23 Trigonometric Ratios of Standard Angles ICSE Solutions Class 9 Maths

ICSE Solutions for Selina Concise Chapter 23 Trigonometrical Ratios of Standard Angles Class 9 Maths

Exercise 23(A)

1. find the value of:

(i) sin 30^o cos 30^o

(ii) tan 30^o tan 60^o

(iii) cos²60^o + sin²30^o

(iv) cosec²60^o – tan²30^o

(v) sin²30^o + cos²30^o + cot²45^o

(vi) cos²60^o + sec²30^o + tan²45^o.

Answer

2. find the value of :

(i) tan² 30^o + tan² 45^o + tan² 60^o

(ii) tan45°/cosec30° + sec60°/cot45° - 5sin90°/2cos0°

(iii) 3 sin² 30^o + 2 tan² 60^o – 5 cos² 45^o.

Answer 

3. Prove that:

(i) sin 60^o cos 30^o + cos 60^o. sin 30^o = 1

(ii) cos 30^o. cos 60^o – sin 30^o. sin 60^o = 0

(iii) cosec² 45^o – cot² 45^o = 1

(iv) cos² 30^o – sin² 30^o = cos 60^o.

(v) [(tan60° + 1)/(tan60° + 1)]² = (1+cos30°)/(1-cos30°)

(vi) 3 cosec² 60^o – 2 cot² 30^o + sec² 45^o = 0.

Answer

4. Without using table prove that:

(i) sin (2 × 30^o) = 2tan30°/(1+tan² 30°)

(ii) cos (2 × 30^o) = (1-tan² 30°)/(1+tan² 30°)

(iii) tan (2 ×30^o) = 2tan30°/(1 - tan² 30°)

Answer

5. ABC is an isosceles right-angled triangle. Assuming of AB = BC = x, find the value of each of the following trigonometric ratios:

(i) sin 45^o

(ii) cos 45^o

(iii) tan 45^o

Answer

6. Prove that:

(i) sin 60^o = 2 sin 30^o cos 30^o.

(ii) 4 (sin⁴ 30^o + cos⁴ 60^o) -3 (cos² 45^o – sin² 90^o) = 2

Answer

7. (i) If sin x = cos x and x is acute, state the value of x.

(ii) If sec A = cosec A and 0^o ≤ A ≤ 90^o, state the value of A.

(iii) If tan θ = cot θ and 0^o ≤ θ ≤ 90^o, state the value of θ.

(iv) If sin x = cos y; write the relation between x and y, if both the angles x and y are acute.

Answer

8. (i) If sin x = cos y, then x + y = 45^o ; write true of false.

(ii) secθ. cotθ = cosecθ; write true or false.

(iii) For any angle, state the value of : sin²θ+ cos²θ

Answer

9. State for any acute angle whether:

(i) sin θ increases or decreases as θ increases:

(ii) cos θ increases or decreases as θ increases.

(iii) tan θ increases or decreases as θ decreases.

Answer

(i) For acute angles, remember what sine means: opposite over hypotenuse. If we increase the angle, then the opposite side gets larger. That means “opposite/hypotenuse” gets larger or increases.
(ii) For acute angles, remember what cosine means: base over hypotenuse. If we increase the angle, then the hypotenuse side gets larger. That means “base/hypotenuse” gets smaller or decreases.
(iii) For acute angles, remember what tangent means: opposite over base. If we decrease the angle, then the opposite side gets smaller. That means “opposite/base” gets decreases.

10. If √3 = 1.732, find (correct to two decimal place) the value of each of the following:

(i) sin 60^o

(ii) 2/tan 30°

Answer

11. Evaluate:

(i) (cos 3A - 2cos 4A)/(sin 3A + 2sin 4A), when A = 15^o.

(ii) [3 sin 3B + 2cos(2b + 5°)]/[2 cos3B - sin(2B - 10°); when B = 20^o.

Answer

Exercise 23(B)

1. Given A = 60^o and B = 30^o, prove that:

(i) sin (A + B) = sin A cos B + cos A sin B

(ii) cos (A + B) = cos A cos B – sin A sin B

(iii) cos (A – B) = cos A cos B + sin A sin B

(iv) tan (A – B) = (tan A - tan B)/(1+tan A.tan B)

Answer

2. If A =30^o, then prove that:

(i) sin 2A = 2sin A cos A = 2tanA/(1+tan²A)

(ii) cos 2A = cos²A – sin²A = (1-tan²A)/(1+tan²A)

(iii) 2 cos² A – 1 = 1 – 2 sin²A

(iv) sin 3A = 3 sin A – 4 sin³A.

Answer

3. If A = B = 45^o, show that:

(i) sin (A – B) = sin A cos B – cos A sin B

(ii) cos (A + B) = cos A cos B – sin A sin B

Answer

4. If A = 30^o; show that:

(i) sin 3 A = 4 sin A sin (60^o – A) sin (60^o + A)

(ii) (sin A – cos A)² = 1 – sin 2A

(iii) cos 2A = cos⁴ A – sin⁴ A

(iv) (1- cos2A)/sin2A = tan A

(v) (1+sin2A + cos2A)/(sin A+ cos A) = 2 cos A.

(vi) 4 cos A cos (60^o – A). cos (60^o + A) = cos 3A

(vii) (cos³ A - cos 3A)/cosA  + (sin³ A - sin3A)/sinA  = 3

Answer

Exercise 23(C) 

1. Solve the following equations for A, if :

(i) 2 sin A = 1

(ii) 2 cos 2 A = 1

(iii) sin 3 A = √3/2

(iv) sec 2 A = 2

(v) √3 tan A = 1

(vi) tan 3 A = 1

(vii) 2 sin 3 A = 1

(viii) √3 cot 2 A = 1

Answer

(iv)

2. Calculate the value of A, if :

(i) (sin A – 1) (2 cos A – 1) = 0

(ii) (tan A – 1) (cosec 3A – 1) = 0

(iii) (sec 2A – 1) (cosec 3A – 1) = 0

(iv) cos 3A. (2 sin 2A – 1) = 0

(v) (cosec 2A – 2) (cot 3A – 1) = 0

Answer

3. If 2 sin x^o – 1 = 0 and x^o is an acute angle; find :

(i) sin x^o

(ii) x^o 

(iii) cos x^o and tan x^o.

Answer

4. If 4 cos²x^o – 1 = 0 and 0 ≤ x^o ≤ 90^o, find:

(i) x^o 

(ii) sin² x^o + cos² x^o

(iii) 1/cos² x° - tan² x°

Answer

5. If 4 sin²θ- 1= 0 and angle is less than 90^o, find the value of and hence the value of cos²θ + tan²θ.

Answer

6. If sin 3A = 1 and 0 A 90^o, find:

(i) sin A 

(ii) cos 2A

(iii) tan²A – 1/cos² A

Answer

sin 3A = 1 
⇒sin 3A = sin90°
⇒ 3A = 90°
⇒ A = 30°

7. If 2 cos 2A = √3 and A is acute, find:

(i) A 

(ii) sin 3A

(iii) sin² (75^o – A) + cos² (45^o +A)

Answer

8. (i) If sin x + cos y = 1 and x = 30^o, find the value of y.

(ii) If 3 tan A – 5 cos B = √3 and B = 90^o, find the value of A.

Answer

9. From the given figure, find:

(i) cos x^o

(ii) x^o

(iii) 1/tan²x° - 1/sin²x°

(iv) Use tan x^o, to find the value of y.

Answer

10. Use the given figure to find:

(i) tan θ^o

(ii) θ^o 

(iii) sin²θ^o – cos²θ^o

(iv) Use sinθ^o to find the value of x.

Answer

11. Find the magnitude of angle A, if:

(i) 2 sin A cos A – cos A – 2 sin A + 1 = 0

(ii) tan A – 2 cos A tan A + 2 cos A – 1 = 0

(iii) 2 cos² A – 3 cos A + 1 = 0

(iv) 2 tan 3A cos 3A – tan 3A + 1 = 2 cos 3A

Answer

12. Solve for x:

(i) 2 cos 3x – 1 = 0

(ii) cos = 0

(iii) sin (x + 10^o) = 1/2 

(iv) cos (2x – 30^o) = 0

(v) 2 cos (3x – 15^o) = 1 

(vi) tan² (x – 5^o) = 3

(vii) 3 tan² (2x – 20^o) = 1

(viii) cos [(x/2) + 10] =√3/2

(ix) sin² x + sin² 30^o = 1

(x) cos² 30^o + cos² x = 1

(xi) cos² 30^o + sin² 2x = 1

(xii) sin² 60^o + cos² (3x- 9^o) = 1

Answer

13. If 4 cos² x = 3 and x is an acute angle; find the value of :

(i) x 

(ii) cos² x + cot² x

(iii) cos 3x 

(iv) sin 2x

Answer

14. In ABC, B = 90^o, AB = y units, BC = units, AC = 2 units and angle A = x^o, find:

(i) sin x^o

(ii) x^o 

(iii) tan x^o

(iv) use cos x^o to find the value of y.

Answer 

15. If 2 cos (A + B) = 2 sin (A – B) = 1; find the values of A and B.

Answer 15: